Frequency Analysis of Sound Waves

Theory

Sound waves can be analyzed in terms of their amplitude and frequency. The loudness of a sound corresponds to the amplitude of the wave, and is measured in decibels. The frequency of a sound wave affects the pitch of the sound we hear. Most musical sounds are composed of a superposition of many frequencies called partial tones, or simply partials. The lowest frequency for a given sound is called the fundamental frequency. For a vibrating object like a tuning fork, this is also the natural resonant frequency of the sound source. Partial tones that are whole multiples of the fundamental frequency are called harmonics. A tone that has twice the frequency of the fundamental is called the second harmonic, which is one octave higher than the fundamental. For example, middle C in the equal-tempered chromatic scale has a pitch of 262 Hz, and the same note one octave higher has a frequency of 524 Hz. The combination of multiple frequencies is what gives a sound its characteristic quality. In this lab, you will analyze the sounds from several different sources and examine the qualities that make each sound unique.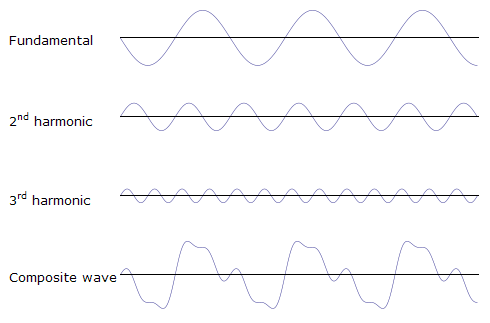

Figure 1

Note	Letter name	Frequency (Hz)	Frequency ratio	Interval
do	C	264	9/8 10/9 16/15 9/8 10/9 9/8 16/15	Whole Whole Half Whole Whole Whole Half
re	D	297
mi	E	330
fa	F	352
sol	G	396
la	A	440
ti	B	495
do	C'	528
Table 1: Diatonic C Major scale

Note

Frequency
(Hz)

Frequency
ratio

Interval

262

Half

C♯ or D♭

277

294

D♯ or E♭

311

330

349

F♯ or G♭

370

392

G♯ or A♭

415

440

A♯ or B♭

466

494

524

Table 2: Equal-tempered chromatic scale

Resonance

When two waves of the same wavelength encounter each other by traveling in opposite directions in the same medium, they combine under certain conditions to yield standing waves. Standing waves are patterns whose points of maximum and minimum (zero) amplitude are fixed so that, apart from a rapid vibration of the medium, the pattern appears to be standing still. If a string is fixed at both ends, each wave on the string will be reflected every time it reaches either end of the string. In general, the multiply-reflected waves will not all be in phase, and the amplitude of the wave pattern will be small. However, at certain frequencies of oscillation, the reflected waves are in phase with the incoming waves, resulting in a standing wave with a large amplitude. These frequencies are called resonant frequencies.

Standing Waves on a String

As stated earlier, if a string is fixed at both ends, then each end must be a node (a point of no displacement). The simplest standing wave pattern that occurs is shown below (called the fundamental mode of oscillation), along with two other modes. The wavelength of the fundamental mode is twice the string length.

Figure 2

Standing Waves in a Half-Open Tube

The standing waves produced by sound waves in air in a half-open tube are longitudinal waves with a node at the interface between media where reflections take place (between water and air in this experiment) and an antinode at the open end of the tube. Patterns of the fundamental (first harmonic) and third harmonic modes of oscillation are shown in the figure below. (Only odd harmonics appear in a half-open tube due to the conditions that there must be a node at the closed end and an antinode at the open end.) From the figure, we see that in the length L from the open end of the tube to the reflective surface, there are an odd number of quarter wavelengths. This can be expressed as:

( 1 )

L = (n/4)λ, where n = 1, 3, 5 ...

Figure 3

Procedure

Part A. Waveform Analysis with the computer interface

Throughout this lab, you will use a microphone and Vernier LabPro interface connected to your CCI laptop to collect sound data, and the Vernier LoggerPro software to analyze the sound waves with frequencies that range from 20 Hz to 16,000 Hz (approximately the range that humans can hear).

1
Connect the LabPro interface to a USB port on your CCI laptop computer.
2
Make sure the power cable is connected to the LabPro interface.
3
Connect the microphone to one of the ports (i.e. CH 1) on the LabPro interface.
4
Start the LoggerPro software. (If this program is not already installed on your computer, it can be downloaded from the Physics 24 lab website.) An experiment window with a graph of Sound Pressure versus Time should appear automatically.
5
Click on the Collect button to begin recording data. Hum into the microphone while the computer collects data. A sound wave should appear if everything is working properly.
6
To examine the frequency spectrum of this data, Insert an FFT Graph from the Additional Graph menu. Use the cursor to identify the most prominent frequencies. The frequency (in Hz) is indicated by the first number in the lower left-hand corner of the graph.
7
Depending on the frequencies that you are examining, you may need to adjust the Sampling Rate or the length of time that data is collected. These settings can be modified by clicking on the stopwatch icon in the experiment window or by selecting Data Collection under the Experiment menu. A setting that should work for analyzing most sounds is 10,000 samples/sec for a period of about 0.05 seconds. Note: If the settings require more than 12,000 data points, the interface will be overloaded.

Part 1. Tuning Fork

1
Strike a tuning fork on the green rubber holder or with a rubber mallet. Hold the fork up to the microphone and adjust the software settings to observe the sound waveform, which should look like a sine wave. Describe what happens to the amplitude and frequency of the wave as the sound fades away.
2
Repeat the sound analysis using the frequency spectrum that should show a distinct peak at the fundamental frequency. Record this value and compare with the frequency that is stamped on the fork. Do they agree? Which do you think is more accurate? (The frequency on the fork should be accurate to within one or two Hertz, which is about the smallest difference in pitch that our ears can distinguish, and the software should be accurate to within 1%.)
3
Gently strike the tuning fork with the plastic mallet and observe the sound wave and FFT graphs. Record the frequencies of any higher peaks that appear and compare with the fundamental frequency. Describe the quality of the sound produced by striking the tuning fork with the plastic mallet versus the rubber holder. Why does the fork sound different depending on how it is struck?

Part 2. Mono chord

1
Set up the mono chord apparatus and observe the sound waveform and frequency spectrum when the chord is plucked versus hammered.
2
Tune the mono chord to the same note as a guitar or violin string and record any differences you observe. How does the body of the guitar or violin affect the quality of the sound? What evidence do you have to support your claim?

Part 3. Human voice

1
Observe the waveform produced by singing a sustained "ah" and "oh" into the microphone.
2
Observe changes in frequency while increasing the pitch of your voice.
3
Speak or make other sounds in the microphone and observe the results.

Part 4. Theme and Variations

1
Analyze sounds from other musical instruments that are available, or create your own experiment to further investigate the different properties of sound waves.
2
Discuss your findings with other students in the class.

Part B. Standing Waves in an Open Tube

In this investigation, a tuning fork will be used to produce a standing wave in a half-open tube that can be varied in length. The resonance tube will consist of a tall vertical cylinder whose effective length can be changed by changing the depth of water in it. When the vibrating fork is held over a tube of the proper length, the incident waves and those reflected at the opposite end will establish a standing wave. When this situation occurs, an observer nearby will detect a marked amplification of the sound.

1
Find a tuning fork with a fundamental frequency (stamped on the fork) of approximately 500 Hz.
2
Strike the tuning fork on a rubber holder or your knee. Hold the vibrating fork perpendicular to the tube and about an inch above it, but do not allow the fork to touch the glass tube to avoid breaking it!
3
Raise the smaller diameter inner tube along with the vibrating fork and listen for the sound to become louder when resonance is achieved. Record the length of the inner tube that is above the water reservoir. Repeat this procedure to find at least one more resonance length.

Calculate the wavelength using equation 1. From your calculated wavelength of the wave produced, and the known frequency of the fork, compute the velocity of the sound wave using v = fλ. Compare with the value predicted by the equation for the velocity of sound waves in air:

( 2 )

v = (1.4RT/M)^1/2

where R is the universal gas constant (8.31 J/mol · K), M is the approximate molecular weight of air (0.029 kg/mol for dry air, ~0.0287 kg/mol for humid air) and T is the absolute temperature of the air in Kelvin. Record the room temperature.

Discussion

What differences in waveforms did you observe for the monochord and tuning forks? For the human voice? The complexity of the waveform for the voice reveals why it is so difficult to reproduce. How did your empirical value for the speed of sound compare with the predicted value? What other phenomena did you observe?