Equilibrium of Forces Acting at a Point

Introduction

Addition of Forces

Forces are one of a group of quantities known as vectors, which are distinguished from regular numbers (know as scalars) by the fact that a vector has two quantities associated with it, a magnitude and a direction (related to a coordinate axes of the system you are dealing with). These properties completely characterize a vector. A vector may alternatively be described by specifying its vector components. In the case of the Cartesian coordinate system (the system we will be primarily dealing with) there are two components, the x-component and y-component. These two properties also completely characterize a vector. Vectors, and in the case of this lab, force vectors, can be represented pictorially (see Fig. 1) by an arrow pointing in the direction of action of the force, with a length proportional to the strength (magnitude) of the force.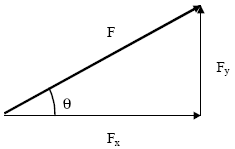

Figure 1

The components

F_x

and

F_y

in the x and y directions of the vector F are related to the magnitude F and angle θ by:

( 1 )

F_x = F cos θ and F_y = F sin θ

and conversely:

( 2 )

F =

F_x² + F_y²

, and θ = arctan

F_y

F_x

When several forces act on a point, their sum can be obtained according to the rules of vector algebra. Graphically, the sum of two forces

= F

₁ + F

₂

can be found by using the parallelogram rule illustrated in Fig. 2 or, equivalently, by the head-to-tail method illustrated in Fig. 3.

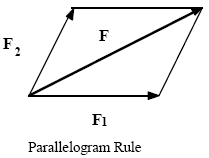
Figure 2

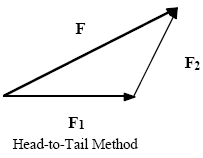
Figure 3

The sum of the vectors can also be derived analytically by adding their components:

( 3 )

F_x = F_1x + F_2x, and F_y = F_1y + F_2y

Condition for Translational Equilibrium

An object is in translational equilibrium when the vector sum of all the forces acting on it is zero. In this experiment we shall study the translational equilibrium of a small ring acted on by several forces on an apparatus known as a force table, see Fig. 4. This apparatus enables one to cause the forces of gravity acting on several masses (F = mg) to be brought to bear on the small ring. These forces are adjusted until equilibrium of the ring is achieved. You will then add the forces analytically by adding their components and graphically by drawing the vectors and determining if they add to zero using the rules for the addition of force vectors listed above.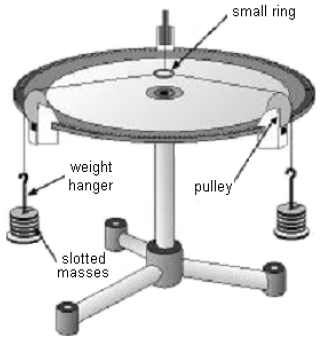

Figure 4

Procedure

Equilibrium with Three Forces

We shall first study the equilibrium of the small ring when there are three forces acting on it. Two of the forces

₁ and F

₂)

will be fixed and the third one

₃

adjusted until equilibrium is reached.

1
If necessary, level the force table using the small bubble level placed on the table's surface.
2
Choose any two masses you like in the range 100-300 g, and place each mass on a weight holder. Use the electronic balance to measure each of the masses including the holder. Designate the measured masses as
m₁ and m₂.
The uncertainty of these measurements should be limited to the precision of the balance.
3
Place the pin in the middle of the force table and place the ring over the pin. Attach two of the four pulleys provided to the force table at any position other than zero degrees. Record the value of θ₁ and θ₂. The uncertainty in these angles should be limited to the precision to which you can read the angles on the force table.
4
Run two of the strings (attached to the ring) over the pulleys, and suspend the masses that you have chosen at the appropriate angles
(m₁ at θ₁ and m₂ at θ₂).
The tension in the two strings acts on the ring with forces
F₁ and F₂,
each with a magnitude equal to the weight of the corresponding mass and holder
(m₁g and m₂g)
suspended at the end of each of the strings.
5
Pull one of the remaining strings in various directions until you locate a direction in which the ring is freed from the pin when you apply the right amount of force. Attach a third pulley at this position. Run the string over the pulley and attach a weight holder to the string. Add weights to the weight holder until the ring pulls away from the pin, so that the pin is not necessary to hold the ring in place. This last added force is the (equilibriant) force F₃
(m₃g).
It may be necessary to make minor adjustments to the angle to obtain a precise measurement. Make sure that the strings are stretched radially and the pin is at the center of the ring. Estimate the uncertainty in the equilibrant force by adjusting the mass and angle until the system is no longer in equilibrium.

Equilibrium with Four Forces

1
Now select three masses (to provide three forces with sum
F₁ + F₂ + F₃)
at three angles (one of them zero) and determine what fourth single mass and angle establishes equilibrium on the force table (the equilibriant force
F₄).
2
Record all angles, masses and their uncertainties as in Equilibrium with Three Forces.

Be sure to pledge your work, initial your data, and have your TA initial your data.

Analysis

Graphical Analysis

Make accurate diagrams on normal rectangular graph paper showing the sum of the forces acting on the ring for both parts of the experiment above.

1
Draw force diagrams to scale. For example, 5 Newtons = 1 cm. Use whatever works best to give you the greatest plotting precision.
2
Use the head-to-tail method to find the sum of the forces graphically. Be as accurate as possible. Qualitatively verify that the sum is zero. If it is not, determine from your graph the magnitude of the deviation from zero.

Analytical Sum

Calculate the resultant force on the ring,

_T = F

₁ + F

₂ + F

₁,

analytically for Equilibrium with Three Forces only. Choose zero degree to be the +x-axis, and 90° to be the +y-axis. The Analytical Sum section of the WebAssign question for this lab, will help facilitate the error analysis.

1
Use the tables in the WebAssign question to enter the data for the forces acting on the ring. For each force, include the magnitude F, its uncertainty u_F, the direction θ, and its uncertainty
u_θ.
The values of
u_θ
must be expressed in units of radians.
2
Calculate the x- and the y-components of each of the forces together with their errors. Pay attention to the sign of each component. Include in the last row of the table the sum of the components and their error.
3
Calculate the magnitude and the direction of the resultant force
F_T.
Compute also the uncertainty of the magnitude.

Discussion

1
Is the condition for static equilibrium satisfied for both parts of this experiment? Remember to consider the experimental uncertainty when answering this question.
2
What do you believe is the primary source of uncertainty in this experiment?
3
Discuss attempts that you made (or should have made) to reduce both systematic and random errors in this experiment.