Print

Thin-Film Interference

Introduction

Interference occurs when the light of wavelength, λ, from two coherent sources arrives at the same point. Let
r1
 be the length of the path taken by the light from one source to the observation point and let
r2
 be the length of the path taken by the light from the other source to the observation point, so that
|r2 − r1|
 is the path difference. If the path difference is an integer number of wavelengths, the path difference produces a zero phase difference at the observation point:
( 1 )
|r2 − r1| = mλ, where m = 0, 1, 2, ... gives zero phase difference.
If the path difference corresponds to a half-integer number of wavelengths, the path difference produces a 180° (half-cycle) phase difference at the observation point:
( 2 )
|r2 − r1| = (m +
1
2
)λ, where m = 0, 1, 2, ... gives 180° phase difference.
A phase difference of zero means that the two waves interfere constructively, producing a resultant wave of larger amplitude. For a phase difference of 180° the two waves interfere destructively, producing a resultant wave of small amplitude. For electromagnetic waves, the amplitude of the wave determines the intensity of the light, so in an interference pattern, constructive interference corresponds to a bright region and destructive interference corresponds to a dark region. One application of interference is reflection from a thin film, where the interference is between light reflected at the top and at the bottom of the film, as shown in Figure 1. For light incident perpendicular to the film (normal incidence), the path difference for the two rays 1 and 2 is 2t, where t is the thickness of the film.
Two horizontal parallel lines. A vertical line with arrows at each end labeled t indicates the distance between the horizontal lines. From the top left of the figure going downward and toward the right to the top horizontal line and then going upward and toward the right at the same angle indicates the ray reflected off the top surface. This ray is labeled 1. Between the parallel lines, A V shaped line goes from the point of reflection at the top surface to the bottom horizontal line and then up and to the right to the top surface. This V represents the ray within the material reflecting off the bottom surface. The slope of the V lines are slightly steeper than the lines representing ray 1. Where the right end of the V meets the top surface, the ray exits the top surface with a slightly less steep slope and continues upward and to the right. This line represents the ray that went into the material, reflected from the bottom surface and exited the material and is labeled 2.
Figure 1
To determine the phase difference produced by the path difference, the path difference must be compared to the wavelength,
λn
, of the light in the film where
λn = λair/n,
λair
 is the wavelength of the light in air, and n is the refractive index of the film material. But there can also be a 180° phase change, or not, in the reflection process. Let light traveling in a material of refractive index n1 reflect from the surface of a material of refractive index n2. If
n1 > n2
, there is no phase change due to the reflection whereas if
n1 < n2
 the reflection produces a 180° phase change. The phase change due to reflection must be combined with the phase change due to the path difference to derive the condition for destructive interference and for constructive interference. The result is the following.
If neither ray has a phase change due to reflection or if both have a phase change then
2t = mλn, m = 0, 1, 2, ... gives constructive interference
2t = (m +
1
2
)λn, m = 0, 1, 2, ... gives destructive interference.
If only one of the rays has a phase change due to reflection then
2t = mλn, m = 0, 1, 2, ... gives destructive interference
2t = (m +
1
2
)λn, m = 0, 1, 2, ... gives constructive interference.

Objective

In this lab we will analyze thin-film interference data from an experiment done with a thin air wedge between two glass plates. You will use this data to determine the thickness of a human hair.

Apparatus

  • Paper
  • Calculator
  • Pencil

Procedure

Please print the worksheet for this lab. You will need this sheet to record your data.

PDF file

Data

You will be given representative data from the following experiment and will be asked to analyze it. Two very flat glass plates, each 12.0 cm long are one on the top of the other. A human hair with diameter, d, is placed between the plates so that a thin wedge of air of varying thickness is created between the two plates, as shown in Figure 2. At a distance, x, from the end of the plates where they are in contact the thickness of the air wedge is t.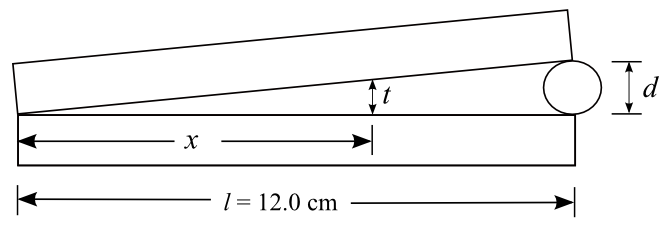
The figure shows two rectangles. The first rectangle lies flat horizontally. An arrow labeled l = 12.0 cm is positioned directly under the rectangle and runs its full length. Another arrow labeled x runs from the left edge of the rectangle to approximately three quarters of the length. A second rectangle is inclined and touches the horizontal rectangle on the left. Two vertical arrows show the distance between the rectangles at different locations. A vertical arrow labeled t runs between the rectangles above the end of t he x arrow. A vertical arrow labeled d runs between the rectangles above the end of the 8 cm arrow. There is a circle between the rectangles at their right end just touching the bottom of the upper rectangle and the top of the lower rectangle.
Figure 2
Light of wavelength 589.3 nm in air from a sodium vapor lamp is directed at normal incidence onto the plates from above. A series of bright and dark bands (fringes) is observed in the reflected light, due to interference between the light reflected at the top and at the bottom of the air wedge. A measuring microscope is used to examine the interference pattern. It is found that the average distance between the centers of adjacent dark fringes is Δx mm.
Note:
You will be given a value for Δx in the WebAssign question.

Analysis

1
Consider a ray of light reflecting at the top of the air wedge and a ray reflecting at the bottom of the wedge. For light at normal incidence the path difference for these two rays is 2t. Taking into consideration the phase changes that take place upon reflection, which of the following is the condition for destructive interference of the reflected light?
  • •
    2t = mλair, m = 0, 1, 2, ...
  • •
    2t =
    m +
    1
    2
    λair, m = 0, 1, 2, ...
2
Considering similar triangles in Figure 2 leads to the equation
t
x
 =
d
l
. Apply the appropriate condition for destructive interference to relate
λair
 and t for the mth and (m + 1)th dark fringe.
3
Combine these equations with
t
x
 =
d
l
 to obtain equations that give the location x for each of these fringes in terms of m,
λair
, l and d.
4
Use these equations to solve for Δx, the separation between adjacent dark fringes.
5
Use the measured value of Δx that you have been given to solve for the thickness, d, of the hair and record your result.

Copyright © 2013-2014 Advanced Instructional Systems Inc. and Texas A&M University. Portions from North Carolina State University. | Credits