The Reflection and Refraction of Light

Introduction

A transparent material is characterized by its refractive index, n, where

n =

In this equation c is the speed of light in vacuum and is equal to 3.00 × 10⁸ m/s, and v is the speed of light in the material. Since n is a ratio of two speeds, it is a dimensionless quantity. Light travels slower in any material than it does in vacuum, so

n > 1.

The refractive index of air is only very slightly greater than 1 and in this experiment we will take it to be equal to 1.00. The slower light travels in a material, the larger the value of n for that material.

Law of Reflection

When light is reflected from a plane (flat) surface, the angle of incidence (θ_a) is equal to the angle of reflection (θ_r). That is,

θ_a = θ_r

. These angles are measured relative to the normal to the surface.

Law of Refraction

When light passes from one medium into another in which its velocity is different, the direction the ray is traveling changes. This is called refraction and occurs at the interface between the two materials. The angle, θ_a, that the incident ray in material a makes with the normal to the interface and the angle, θ_b, that the refracted ray in material b makes with the normal are related by Snell's law.

( 1 )

n_a sin θ_a = n_b sin θ_b

If light refracts into a material of larger refractive index

(n_b > n_a),

then

θ_b < θ_a

and the ray is bent toward the normal. If light refracts into a material of smaller refractive index

(n_b < n_a),

then

θ_b > θ_a

and the ray is bent away from the normal.

Critical Angle and Total Internal Reflection

If a ray refracting from a slow medium that has refractive index, n_a, into a faster medium that has smaller index of refraction, n_b, has an angle of incidence such that

θ_b = 90°,

then the ray is refracted along the interface between the two media. Under those conditions, θ_a is called the critical angle (θ_c). Furthermore, if

θ_a > θ_c,

then Snell's law requires that

sin θ_b > 1.

This is not possible, so for

θ_a > θ_c

there is no refracted ray and all the incident light is reflected back into medium a. This is called total internal reflection. Total internal reflection can occur only when

n_a > n_b,

that is when the light has smaller velocity in the incident material. The condition that

θ_b = 90°

when

θ_a = θ_c

gives that

( 2 )

sin θ_c =

n_b

n_a

Image Formation By Reflection at a Flat (Plane) Surface

After they reflect from a flat mirror surface, rays from a point object appear to come from a point behind the mirror. This point is the virtual image of the object. The law of reflection can be used to show that the virtual image is the same distance behind the mirror as the object is in front of the mirror.

Objective

In this lab we will verify the law of reflection and locate the virtual image formed by a flat mirror. We will use Snell's law for refraction to measure the refractive index of a glass plate and to analyze the phenomena of total internal reflection.

Apparatus

Optical bench
Incandescent light source
Ray table and base
Slit plate, slit mask and holder
Transparent square plate
Transparent triangular plate
Plane mirror
Protractor
White paper, masking tape and scissors

Procedure

Please print the worksheet for this lab. You will need this sheet to record your data.

Setup

The basic ray optics setup is shown in Figure 1.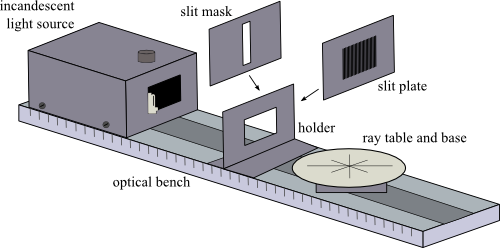

Figure 1

The ray table is placed over the pin protruding from the top of the ray table base, which is positioned flush against the alignment rail on the optical bench. Note that the ray table base is designed such that it tilts slightly towards the incandescent light source. This will ensure that the images on the ray table appear bright and sharp. The errors in your readings due to this tilt are negligible. There is a parallel ray lens attached to the front of the light source. It will help in keeping the rays parallel and should be left on the source during the experiment.

Place the component holder on the bench between the light source and the ray table, against the alignment rail. Attach the slit plate on the holder facing the ray table and attach the slit mask on the other side of the holder.

The slit plate will be used to block out all but one single ray. The distance between the light source and the slit plate/mask combination may be adjusted for the best sharpness and clarity, but there is a corresponding loss of brightness with distance.

Place a circular piece of white paper on the ray table so that it covers it and tape it into place.

Caution:
Do not mark on the ray table.

A single ray of light can be obtained on the ray table (and made sharp and visible) by following these steps (or a combination of these steps):

a
adjusting the lateral position of the slit plate
b
adjusting the position of the filament with the filament knob placed on top of the incandescent light source
c
adjusting the position of the ray table
d
positioning the slit mask

Law of Reflection

Place the ray optics mirror on the paper circle so that a single light ray shines on the flat side of the mirror and is reflected. Draw a line on the paper that is along the flat side of the mirror.

Rotate the ray table slowly until the ray from the light source makes an angle of about 45° with the normal to the mirror surface. Mark the positions of the incident and reflected rays by marking two points along each ray (Figure 2). Remove the sheet from the ray table and trace the path of the incident and reflected rays by joining the two points of each ray. At the point where the two rays touch the mirror surface, draw the normal.

Figure 2

Use a protractor to measure the angles of incidence (θ_a) and reflection (θ_r) with respect to the normal to the mirror surface and record these values.

Which of the following statements best represent your data?

•
θ_a is much less than θ_r.
•
θ_a is much greater than θ_r.
•
θ_a is equal to θ_r.

Image Formed By a Plane Mirror

Remove the parallel ray lens from the front of the light source. Remove the slit mask from the component holder. There should be multiple light rays shining through the slit plate. Adjust the position of the slit plate for sharp and visible rays. Tape a new paper circle on top of the ray table and place the mirror on top of the paper. The front of the ray table should be about two inches from the light source box. Position the mirror so that all or most of the light rays are reflected from its plane surface and rotate the ray table so that the angle of incidence is about 45°.

Draw a line on the paper to mark the position of the flat surface of the mirror and mark two points along each of the incident and reflected rays. (It is only necessary to mark three of the rays in each group, the two extremes and the middle. Label the points (r₁, r₂, etc.) so that you know which points belong to which ray. (See Figure 3.)

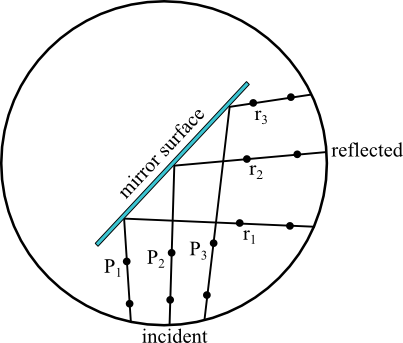
Figure 3

Remove the paper circle and trace the rays as shown in Figure 4. Draw dotted lines to extend the incident and reflected rays. (You may need to tape additional sheets of paper to your paper circle.) The incident rays should trace back to a common point, the location of the filament in the bulb of the light source. The reflected rays should trace back to the virtual image of the filament. Draw a straight dotted line to connect the location of the filament (object) and the image. Extend the line that was along the flat surface of the mirror. It should be perpendicular to the line that connects the object and image locations.

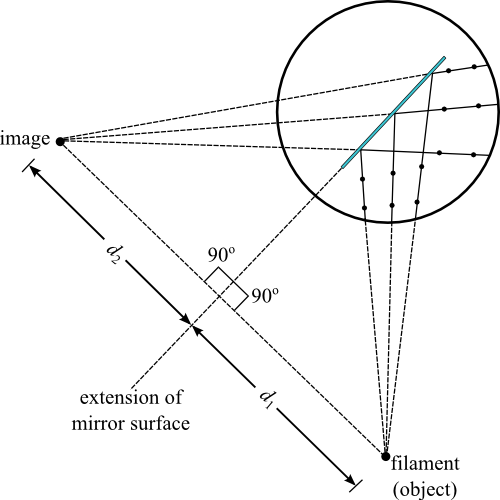
Figure 4

Measure and record the object distance (d₁) and image distance (d₂), as shown in Figure 4.

Which of the following statements best represent your data?

•
d₁ is much less than d₂.
•
d₁ is much greater than d₂.
•
d₁ is equal to d₂.

Measuring n

Put the slit mask back on the holder and put the parallel ray lens back on the opening of the light source box. Tape a paper circle sheet onto the ray table and place the square plastic (or glass) plate on the paper so that the incident ray is at normal incidence. Draw lines along the outline of the square plate to show its position on the paper. Slowly rotate the ray table until the refracted ray is visible on the opposite side of the plate, with an angle of incidence of about 45°. Note that the ray exiting the plate is parallel to the incident ray, but has a lateral displacement.

Figure 5

Mark the positions of the incident and refracted rays with points as before (P₁, P₂, P₃, and P₄ as in Figure 4.) Remove the paper from the ray table. Connect the marked points to draw the incident ray and the ray refracting back into the air at the opposite face of the plate. Draw the normal to the plate face where the ray enters. Draw the line that gives the path of the ray through the plate. With a protractor, measure the angles θ_a and θ_b, the angles of the incident and refracted ray for the ray as it refracts from air into the plate (Figure 5) and record your results.

Use Snell's law with your measured values for θ_a and θ_b and 1.00 for the refractive index of air to calculate the refractive index of the material of the square plate and record your result.

Critical Angle and Total Internal Reflection

With the single ray setup for the light source, tape another circular sheet of paper onto the ray table. Place the triangular prism on the paper so only a single ray is incident normal to one face of the prism and a ray emerges back into the air at the opposite face (Figure 6).

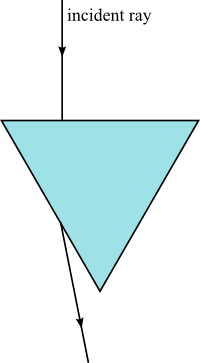
Figure 6

Draw lines on the paper along the outline of the prism. Slowly rotate the ray table counterclockwise, to increase the angle of incidence until the refracted ray disappears. Now rotate the table slightly clockwise until the refracted ray just reappears, parallel to the face. For this orientation of the prism, the angle of incidence of the ray in the prism at the interface with air is the critical angle, θ_c, for total internal reflection (Figure 7). Mark the incident ray from the light source as it first strikes the prism with two points and mark the point where the refracted ray exits the prism.

Figure 7

Remove the paper from the ray table. Connect the two points you drew along the incident ray with a straight line that intersects the surface of the prism. Draw a line between the point where the ray enters the prism to where it exits; this is the path of the ray inside the prism (Figure 8). Draw dotted lines to show the normal to each face, where the ray enters and where it exits the prism. Use a protractor to measure the angle of incidence, θ_a, and the angle of refraction, θ_b, for the ray as it enters the prism and record your results.

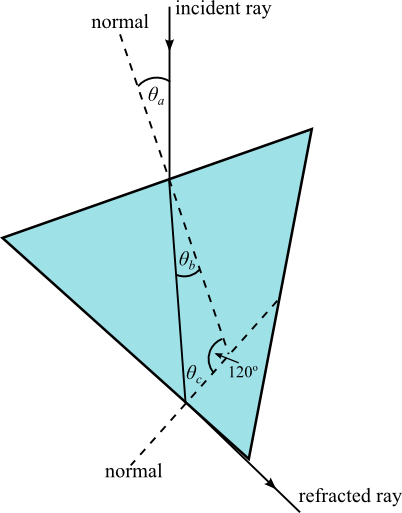
Figure 8

Use Snell's law with your measured values for θ_a and θ_b and 1.00 for the refractive index of air to calculate the refractive index of the material of the prism plate and record your result.

Use your measured value of the refractive index for the prism to calculate the critical angle, θ_c, for a ray traveling in the prism and incident on an interface with air. Record your result.

Figure 8 shows that

θ_b + θ_c = 60.0°.

Record the value of θ_c given by this equation and your measured value of θ_b.

How well does the value of θ_c calculated from n for the prism compare with the value from your measured θ_b?

Before you leave the lab room be sure to remove all pieces of tape from the ray table.