Resistors in Series and Parallel

Introduction

Direct current (DC) circuits are characterized by the quantities current, voltage and resistance. Current is the rate of flow of charge. The SI unit is the ampere (A). By convention the direction of the current is the direction of flow of charge, even though in metallic conductors the current is due to flow of negative charge (electrons) in the opposite direction. Because of conservation of charge, the current is the same at all points in a single loop circuit. At a branch point in a circuit, where the conducting path splits into two or more paths, the total current into a branch point equals the total current out of that point. By convention, current flows out of the positive terminal of a battery or power supply and into the negative terminal. For current to be maintained in a circuit there must be a complete conducting path. Voltage is a measure of the electric potential difference between two points in a circuit. The SI unit is the volt (V). Since the electrical force is a conservative force, the sum of the voltage increases and decreases around any closed loop is zero. Resistance is the property of a circuit element (conductor) to oppose current flow. Resistance is defined by

( 1 )

R =

where V is the voltage across the circuit element and I is the current flowing through it. If R is constant, the same for all V, then the circuit element obeys Ohm's Law. The SI unit of resistance is the ohm (Ω). The resistance of a resistive circuit element changes with temperature. Two resistors R₁ and R₂ are connected in series if all the current that passes through R₁ also passes through R₂. Therefore, for two resistors in series the current I₁ through R₁ is the same as the current I₂ through R₂, and this current is the same as the current, I, that enters the series network:

I = I₁ = I₂.

The total voltage, V, across the series network is the sum of the voltages V₁ and V₂ across each resistor. That is,

V = V₁ + V₂.

The equivalent resistance, R_s, of R₁ and R₂ in series is given by

( 2 )

R_s = R₁ + R₂.

Two resistors R₁ and R₂ are connected in parallel if the voltages V₁ and V₂ across each are the same and equal to the voltage, V, across the parallel network. That is,

V = V₁ = V₂.

The currents I₁ and I₂ through each of the resistors add to give the total current, I, flowing into and out of the network:

I = I₁ + I₂.

The equivalent resistance R_p of R₁ and R₂ in parallel is given by

( 3 )

R_p

R₁

R₂

This can also be written as

( 4 )

R_p =

R₁R₂

R₁ + R₂

Ammeters are used to measure current. An ammeter is connected in series with the circuit so that all the current being measured flows through the ammeter. Therefore, ammeters need to have very small resistance in order not to alter the current in the circuit. Voltmeters are used to measure voltages. A voltmeter is connected in parallel at the two points between which the potential difference is to be measured. Therefore, a voltmeter needs to have a large resistance so that very little current is diverted through it.

Objective

In this lab we will measure and analyze currents and voltages for circuits containing a single resistor and also for two resistors in series and for two in parallel.

Apparatus

0-40 volt DC power supply
12 volt lightbulb and socket
150 and 700 ohm resistors
Digital multimeter

Procedure

Please print the worksheet for this lab. You will need this sheet to record your data.

Measurement of Voltage

The power supply is a source of potential difference (voltage). Locate the DC power supply at your table. Press the POWER ON/OFF button to the ON position. Next, press the RANGE button to the IN (0.85 A) position. This sets the power supply to the 0-35 V/0-0.85 A range. Rotate the voltage and current ADJUST knob counterclockwise. Then set the maximum output current for this experiment by pressing the CC Set button, and while holding it down rotate the current ADJUST knob clockwise until the AMPS display reads 0.30 A. Release the CC Set button. Do not move the current setting knob (CC Set) at any point during the experiment.

The multimeter is a measuring device that is used to measure voltage differences, electrical currents, and electrical resistances. It can measure other electrical properties as well. See Figure 1. At the top of the meter is an LCD (liquid crystal display), in the middle is the Function/Range Switch (dial), and at the bottom are four input sockets.

Note: The meter is particularly sensitive (and prone to blowing a fuse) when using the 200 mA Input Jack (see I in the key for Figure 1.)

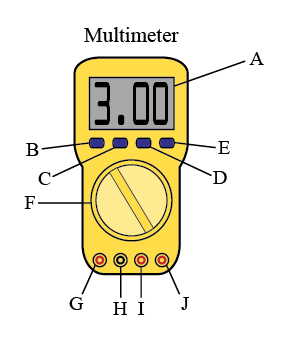
There is a multimeter to the left with a number display that is labeled A. Below the screen are four buttons labeled B through E, then a dial labeled F. Below the dial are four input jacks labeled G through J.

Figure 1

Key for Figure 1:

A
3-1/2 digit LCD with annunciators.
B
ON/OFF button: Turns power to meter on and off.
C
HI/LO button: Selects high or low trigger level for frequency measurements.
D
MAX button: Selects maximum reading hold function.
E
DC/AC button: Selects DC or AC type voltage.
F
Function/range switch: Selects the function and range desired.
G
V Ω Input Jack: Input connector for volts, ohms, diode test, continuity, frequency and logic.
H
COM Input Jack: Ground input connector.
I
200 mA Input Jack: Input connector for current up to 200 mA, L_x (Inductance), C_x (Capacitance).
J
10 A Input Jack: Input connector for current to 10 A.

To measure a given quantity, the dial must be at the appropriate setting and the appropriate two input jacks must be employed. Thus, when turning the dial to change from one type of measurement to another (e.g. from voltage difference to electric current), you may have to change input sockets as well. If overloaded, the fuse can blow out.

Caution:
To protect itself, the meter buzzes when overloaded; if it buzzes, immediately disconnect the meter leads!

To protect the meter from overload when an unknown voltage or current is measured, the meter should first be set to the highest scale for that function. If the reading then is not large enough to give at least three significant figures, the scale should be switched (if possible) to one that permits an accurate measurement.

To turn on the multimeter, toggle the top left button on the meter until there is a display on the meter face. To set up the multimeter to measure DC voltages, V, toggle the top right button to DC. Be sure that the meter display reads DC. Turn the Function/Range Switch to the voltage (V) range and dial the setting to 20. The meter is now set to read voltages up to 20 volts DC. Attach banana to banana leads to the common (COM) jack and to the voltage (V) jack.

Connect the multimeter leads to the + and – terminals of the power supply. See Figure 2. On the power supply, rotate the voltage ADJUST knob clockwise until the volts display reads 5.0 volts. Compare the voltage readings on the multimeter and on the power supply's meter. These two readings may not be exactly the same. The multimeter is expected to be more accurate.

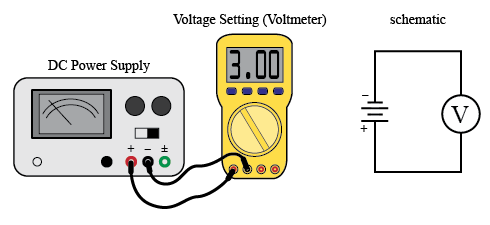
A DC power supply has two wires connecting the positive and negative inputs to a voltmeter. The voltmeter is the same as the multimeter in Figure 1. There is also a schematic of the circuit diagram showing wires connecting the DC power supply to the voltmeter, one from the negative terminal of the power supply to the voltmeter and a second connecting the voltmeter to the positive terminal of the power supply.

Figure 2

Current and Voltage for a Single Resistor

Turn down the voltage of the power supply (counterclockwise) to zero volts. Connect the power supply to the resistor on the circuit board that is marked 700 ohms. (Do not readjust or change the current setting on the power supply.) We will use the multimeter to measure the DC current through the 700-ohm resistor as a function of the applied voltage. To do this, we must connect the multimeter in series with the resistor, so that the same current passes through both. Since it is easy to blow a fuse when the multimeter is on its current setting, follow the instructions carefully. Set the multimeter dial to the 20 mA current scale and connect the banana plugs to the COM and mA jacks on the meter.

Caution:
Do not turn up the voltage on the power supply until your TA checks your circuit.

After being given the go-ahead by your TA, set the power supply to 1 volt and record in Table 1 the current through the resistor as indicated on the multimeter. Repeat with the power supply set to 2, 3, 4, and 5 volts.

Use Excel to plot your data, with current on the vertical axis and voltage on the horizontal axis. Refer to the Appendix in the online lab manual for instructions on graphing with Excel. If you get the results that are expected, the data will fall close to a straight line that passes through the origin. Use Excel to find the slope of the straight line that best fits your data and record your result, including units.

Use Ohm's law and the slope from your graph to calculate the resistance, R, of the resistor, in units of ohms (Ω). Record your result.

Current and Voltage for a Light Bulb

TURN THE POWER SUPPLY VOLTAGE TO ZERO but do not turn off the power supply. Do not readjust the current setting on the power supply (CC Set). We will use the multimeter to measure the DC current through the light bulb as a function of the applied voltage. To do this, we must connect the multimeter in series with the light bulb, so that the same current passes through both. Since it is easy to blow a fuse when the multimeter is on its current setting, follow the instructions carefully.

Set the multimeter dial to the 10 A DC current scale. Use the COM and 10 A input jacks. Connect the circuit as shown in Figure 3.

Caution:
Do not turn up the voltage on the power supply until your TA checks your circuit.

After being given the go-ahead by your TA, turn the power supply voltage to 2 volts. In Table 2 record the current reading on the multimeter. Repeat for power supply voltages of 4, 6, 8, 10, and 12 volts.

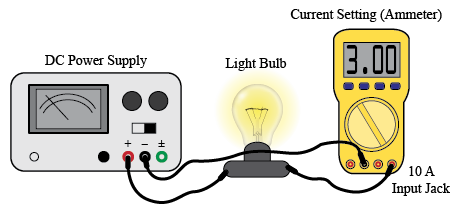
A wire connects the positive input of the DC power supply to a light bulb. A wire connects the other end of the light bulb to a 10 A input jack on an ammeter. The ammeter is the same as the multimeter in Figure 1. A wire then connects the ammeter to the negative input of the DC power supply.

Figure 3

Use Excel to plot your data, with current on the vertical axis and voltage on the horizontal axis. It is expected that your data will not fall close to a straight line. Define

R =

and calculate R for each set of V and I values in Table 2 and record in the third column of Table 2. Does R increase, decrease, or stay the same as the current, I, through the light bulb increases?

Two Resistors in Series

Connect the two resistors on the circuit board that are marked 150 ohm and 700 ohm as shown in Figure 4. They are said to be in series because all the current that passes through one also passes through the other. Make sure the power supply voltage is set to zero. Connect the power supply to the series resistor combination as in Figure 4. Set the power supply to 5 volts. Set the multimeter dial to the 20 V range and use the COM and V jacks. With the multimeter, measure and record the potential differences (voltage) V₁₅₀ and V₇₀₀ across each resistor and the voltage, V, across the two-resistor combination.

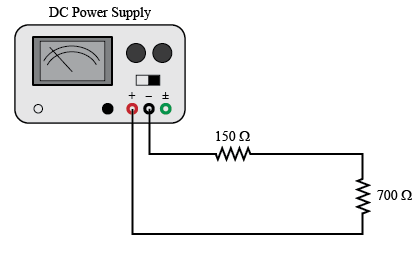
A wire runs from the positive input of the DC power supply to a 700 ohm resistor, followed in series by a 150 ohm resistor, and then back to the negative input of the DC power supply.

Figure 4

When you have completed these measurements, set the power supply voltage to zero and disconnect the multimeter from the circuit. Which of the following better represents your results?

•

V = V₁₅₀ + V₇₀₀
•

V = V₁₅₀ = V₇₀₀

With the power supply voltage set to zero, connect the multimeter in series with the resistors as shown in Figure 5. Set the multimeter to the 200 mA DC current range and connect the multimeter leads to the correct jacks.

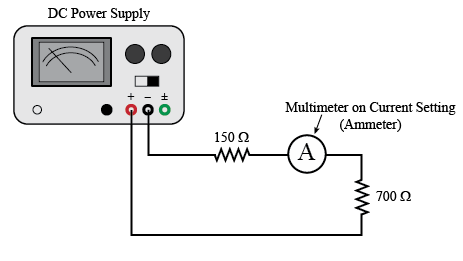
A wire runs from the positive input of the DC power supply to a 700 ohm resistor, followed in series by an ammeter, followed in series by a 150 ohm resistor, and then back to the negative input of the DC power supply.

Figure 5

Caution:
Before proceeding, have your lab instructor check that you have the correct setup: a fuse may blow if the meter is not connected to the proper points in the circuit.

After being given the go-ahead by your TA, turn on the power supply and set it to 5 volts. Measure the current in the wire between the two resistors, the wire between the 700 ohm resistor and the power supply and the wire between the 150 ohm resistor and the power supply. Verify that these three currents are equal.

Continuing with the circuit shown in Figure 5, set the power supply voltage to 2 volts. In Table 3 record the current reading on the multimeter. Repeat for power supply voltages of 4, 6, 8, 10, and 12 volts.

Use Excel to plot your data, with current on the vertical axis and voltage on the horizontal axis. Use Excel to find the slope of the straight line that best fits your data and record your result, including units.

Use Ohm's law and the slope from your graph to calculate the equivalent resistance, R_s, of the two resistors in series, in units of ohms (Ω). Record your result.

Two Resistors in Parallel

Connect the two resistors on the circuit board that are marked 150 ohms and 700 ohms as shown in Figure 6a. They are said to be in parallel since the voltage across each resistor equals the voltage of the power supply and the resistors provide parallel paths for the current flow.

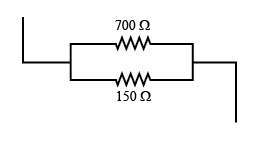
Two resistors of 700 ohms and 150 ohms are shown in parallel.

Figure 6

Make sure the power supply voltage is set to zero. Connect the power supply to the parallel resistor combination as in Figure 6b. Set the power supply to 5 volts. With the multimeter, measure and record the currents I₁₅₀ and I₇₀₀ flowing through each resistor and the total current, I, flowing through the power supply.

A wire runs from the positive input of the DC power supply to two resistors, of 700 ohms and 150 ohms, in parellel, and back to the negative input of the DC power supply.

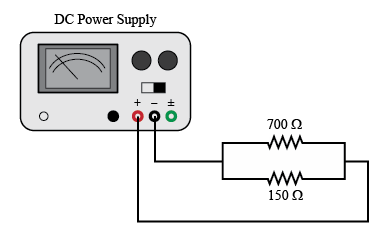
Figure 7

When you have completed these measurements, set the power supply voltage to zero and disconnect the multimeter from the circuit.

Which of the following better represents your results?

•

I = I₁₅₀ + I₇₀₀
•

I = I₁₅₀ = I₇₀₀

Continue with the parallel resistor network. With the power supply voltage set to zero, connect the multimeter to measure the total current, I, flowing through the power supply. Set the multimeter to the 200 mA DC current range.

Caution:
Before proceeding, have your lab instructor check that you have the correct setup: a fuse may blow if the meter is not connected to the proper points in the circuit.

After being given the go-ahead by your TA, turn on the power supply and set it to 2 volts.

In Table 4 record the current reading on the multimeter. Repeat for power supply voltages of 4, 6, 8, 10, and 12 volts.

Use Ohm's law and the slope from your graph to calculate the equivalent resistance, R_p, of the two resistors in parallel, in units of ohms (Ω). Record your result.