Macroscopic and Microscopic Springs – Procedure

Objective

Purpose

In this lab you will:

•
investigate the spring-like properties of a straight wire,
•
discover the "stretchiness" of a material, independent of the size and shape of an object,
•
relate macroscopic "stretchiness" of a material to microscopic "stiffness" of the interatomic bonds.

Some useful equations and constants are given here.

Questions to Explore

1
Is a solid metal, straight wire like a spring? If so, in what ways? If you consider the wire to be a spring, how does spring stiffness compare to the coiled springs used in the previous lab?
2
How can one express the spring-like properties of the particular metal in a way that is independent of the size, shape, and physical dimensions of the wire (Young's modulus)?
3
How can one relate the macroscopic spring-like properties of the particular metal to the stiffness of that metal's interatomic bonds (interatomic spring stiffness).

Equipment

a straight metal wire stretched on a frame, with a hanger for weights
a millimeter scale for determining the position of the end of the wire
Vernier caliper (for how to read — see the Measuring with Vernier Calipers section below)

Procedure

Please print the worksheet for this lab. You will need this sheet to record your data.

Experimental Setup

Recording Data

Right click on the Excel^® file "youngs modulus.xls," and save it to My Documents. Double-click the file to open it in Excel^®.

You should see a display that includes places for your group information, measured values, and calculated values, as well as a space for your graph.

You will record all of your data and analysis on the Excel^® sheet and submit it to WebAssign to be graded by your TA.

Measuring with Vernier Calipers

In this laboratory exercise, you will be using a Vernier caliper, which is comprised of two "teeth," one attached to a fixed scale and the other attached to a sliding (Vernier) scale. In order to measure an object's width, the object is simply placed between the caliper's two teeth. The sliding tooth is then moved until the object is pressed tightly between the teeth. Using both scales, the width can be read to the nearest 0.005 cm (or 0.05 mm). The scales are read as follows (refer to the figure).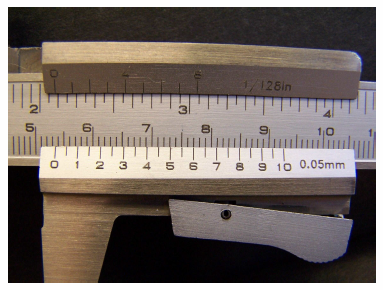

A metal ruler with centimeter and millimeter markings on the bottom and inch and eighth inch markings on the top. Around about a two inch section of this is a larger piece of metal that can slide along the ruler. The top of this piece has markings for 0 to 8 and is labeled 1/128 in. These markings can line up with the markings on the inch side of the ruler. The bottom of the larger piece of metal have markings for 1 to 10 with half markings in between. This side is labeled 0.05 mm and the markings can line up with the centimeter side of the ruler. Extending from the bottom of the larger piece of metal is a handle to make it easier to slide.

Figure 1: Vernier Calipers

Find where the 0 mark of the sliding scale lines up on the fixed scale. In this case, it is just past the 5.3 cm mark. So, the first reading is 5.3 cm.

Find the mark on the sliding scale that most closely lines up with one of the marks on the fixed scale. Here, 5.0 and 6.0 are very close, but 5.5 lines up best with one of the marks on the fixed scale. This value is the number of hundredths of centimeters (or tenths of millimeters). So, the second reading is 0.055 cm.

Add the two values together to get the total reading: 5.3 cm + 0.055 cm = 5.355 cm.

To get more practice reading calipers, visit this website which has an interactive Java applet.

At this website, the label on the sliding scale is misleading. The scale is labeled "mm," which leads the user to think that the numbers on that scale represent millimeters. This is NOT correct. As described above, the numbers on the sliding scale represent tenths of millimeters.

Measurements

Dimensions of the Wire

One of the goals is to describe the "stretchiness" of a material independent of the size and shape of the wire. To do this, we first need to know the physical dimensions of the wire.

Measure the following and enter your data into your Excel^® sheet.

a
Entire length of the wire without any weights on it
b
Diameter of the wire using the caliper (see section above Measuring with Vernier Calipers)
c
Using the measured diameter of the wire, calculate the cross-sectional area of the wire.

Checkpoint:
Compare your data with another group to make sure it is reasonable.

Stiffness of the Wire

To determine if the straight wire behaves like a spring, you need to measure the position of the end of the wire as you add different amounts of weight.

Remove the kinks in the wire.

a
Add 6 kg to the platform hanging from your wire. Remove the 6 kg. Repeat. This helps get small kinks out of the wire, so the "straight" wire doesnt act like a coiled spring.

If the wire has kinks in it, you will measure the springiness of the "coiled" wire instead of the stiffness of a straight wire. Remove the kinks before starting your measurements.

Take the following measurements and record your data in your Excel^® sheet.

a
Look at the scale on the apparatus, and make sure you know what the divisions are and how to read the scale. You should be able to measure to the nearest 0.1 mm.
b
You need to make three sets of measurements, so that you can get average values.
- i
  Take measurements with 0 kg, then 2 kg, 4kg, 6kg.
- ii
  Take all the masses off and start from zero again, taking the same measurements twice more.
- iii
  Take the average of your three sets of measurements.
c
Calculate the variation of your measurement.
- i
  The variation in the measurements (the "reproducibility") gives you an indication of how meaningful the individual measurements are. Record your estimate of the variation.
  - •
    If the measurements are 5.5, 5.7, 5.4, 5.6, 5.8, and 5.6 mm, the average is (5.5 + 5.7 + 5.4 + 5.6 + 5.8 + 5.6)/6 = 5.6, from a minimum of 5.4 to a maximum of 5.8.
  - •
    A compact way to report this average with the approximate variation is 5.6 ± 0.2 mm.
- ii
  If the variation is larger than 0.3 mm, you MUST repeat your measurements.
  - •
    Discard earlier measurements since this was probably getting more kinks out of the wire.

BEFORE graphing your data, discuss the following questions with your group members.

a
If the wire acts like a spring, what would you expect a graph of stretch versus force to look like?
b
How could you determine the spring stiffness of the wire from the graph?

Use your data to make a graph in Excel^® of the stretch of the wire (on the y-axis) versus the force exerted on the wire (on the x-axis).

a
Both of these quantities will need to be computed from your data (either by hand or using Excel^®).
b
From the two columns representing stretch and force, highlight the cells with the data for 2, 4, 6 kg.

Do NOT include the data for 0 kg, because the wire may be kinked without a load.

c
Choose menu option Insert → Chart, and choose 'XY (Scatter).' (Do NOT choose a 'Line' chart.)
d
Be sure to verify that you have the proper quantities on the proper axes.
e
Fit your data to a 'Trendline.'
- i
  Right click on one of the data points and select 'Add Trendline.'
- ii
  Choose 'Linear' under 'Type.'
- iii
  Under 'Options,' choose 'Display Equation on Chart' — this will display the equation of the line that best fits your data in the form
  y = mx + b.
  You can reposition the equation.

f
From your data, determine the spring stiffness of the wire.
- i
  Since
  F = K_ss,
  then
  s =
  1
  k_s
  F,
  so the slope of your graph is equal to
  1/k_s.

Checkpoint:
Compare your measurements, calculations, and graph with another group to verify that they are reasonable.

"Stretchiness" of the Metal

Calculating Young's modulus is a way to measure the "springiness" or "stretchiness" of the metal and factor out the size and shape of the particular wire.

BEFORE calculating Young's modulus, discuss the following questions with your lab partners and write your answers on a whiteboard.

a
If the cross-sectional area of the wire were doubled, would the wire stretch more, less, or the same amount when you hung a 2 kg mass on it? Why?
- i
  Think about putting two of your original wires together side by side.
- ii
  How does this compare to placing two springs in parallel?
b
If the wire were twice as long, would it stretch more, less, or the same amount when you hung a 2 kg mass on it? Why?
- i
  Think about connecting two of your original wires end to end.
- ii
  How does this compare to placing two springs in series?
c
The quantity F/A is called "stress." What are its units?
d
The fractional stretch ΔL/L₀ is called "strain." What are its units?

Checkpoint:
Compare your responses with another group.

Use the measurements for your wire to determine Young's modulus.

a
At equilibrium
F = k_sΔL = s,
so:

( 1 )

Y =

(F/A)

(ΔL/L₀)

(k_sΔL/A)

(ΔL/L₀)

k_sL₀

b
Use your measurement for the initial length, your calculated value for the cross-sectional area, and the value for the spring stiffness obtained from your graph to determine Young's modulus for the material of which your wire is made.
c
Show your calculation on your whiteboard and record your result on your Excel^® sheet.
d
How does your value for Young's modulus compare to that of aluminum (6.2 × 10¹⁰ N/m²) or lead (1.6 × 10¹⁰ N/m²)? Given that the wire is brass (an alloy of copper and zinc), is your value reasonable?

Checkpoint:
Compare your results with another group.

Determining the Interatomic Spring Stiffness

Young's modulus is a way to relate the macroscopic spring-like properties of the particular metal to the stiffness of that metal's interatomic bonds (interatomic spring stiffness).

BEFORE determining the interatomic spring stiffness, discuss the following with your partners.

a
Assuming a simple "ball and spring" model for our solid, how could we determine the length of the interatomic bonds if we knew the density of the material? (Hint: You have probably done something similar in your homework recently.)
b
Using this idea and the following information, determine the length of the interatomic bond for brass.

•
Brass is an alloy of copper and zinc.
•
The atomic mass of copper is 64 grams/mole; zinc is 65 grams/mole.
•
The density of copper is 8.94 grams/cm³, for zinc, it is 7.14 grams/cm³.
•
Note: beware of your units.

Use your calculation of the interatomic bond length and your value for Young's modulus to approximate the stiffness of the interatomic bonds,

k_s,i.

a
In the "Stretchiness" of the Metal section above, we showed:

( 2 )

Y =

k_sL₀

b
For our "ball and spring" model, the cross-sectional area of the bond is A = d² and the relaxed length is L₀ = d (if you are unsure why this is, reread the Interatomic Spring Stiffness section from Macroscopic and Microscopic Springs – Concepts). Thus:

( 3 )

Y =

k_sL₀

k_s,i d

d²

k_s,i

c
Show your calculation on your whiteboard and record your result on your Excel^® sheet.

Checkpoint:
Compare your results with another group.

Submit your Excel^® sheet and answer the follow-up questions in WebAssign.