Conservation of Momentum and Energy

Objectives

•
to investigate simple elastic and inelastic collisions in one dimension to study the conservation of momentum and energy phenomena

Equipment

horizontal dynamics track
collision and dynamics carts with picket fences
photogates connected to the Science Workshop interface

Figure 1

Introduction and Theory

Collisions are an important way of studying how objects interact. Conservation laws have been developed that allow one to say quite a bit about what is happening without knowing the exact details of the interaction during the collision. In this lab, you will show that the total momentum of the system is always conserved when there is no net external force acting on the system, and that the total mechanical energy of the system is only conserved in certain kinds of collisions. These principles are important in studying automobile collisions, planetary motion, and the collisions of subatomic particles. Momentum is the product of mass (m) and velocity (v), so it has the units of kg · m/sec. Momentum is a vector quantity with its direction the same as the velocity. We do not have a special name for the unit of momentum, but we do commonly use the letter p to represent the momentum vector.

( 1 )

p = m · v

Conservation of Momentum is derived in your textbook using Newton's Third Law, and also deals with the quantity called impulse which is force × time, where time is the time interval over which the force acts. In a closed system, momentum is conserved when objects are interacting with each other. Another important conservation law is the Conservation of Mechanical Energy. Energy is a scalar quantity and not a vector. A scalar quantity just has a magnitude and no direction. Mechanical energy is conserved depending on whether the forces between the interacting objects are conservative. Examples of conservative forces are gravity, electric, and magnetic forces. There are other forces at the level of nuclear physics that are also conservative. The most important non-conservative force we will deal with is friction. Friction is a non-conservative force because energy is converted into heat by friction. Another example of a non-conservative "force" will occur when we have two bodies that collide and stick together. This will be a special case of friction where the energy will be converted into heat in the process of sticking together. In this experiment, we will be dealing with collisions in one dimension. The motion of the bodies involved is constrained to a horizontal track. This means that the velocity and momentum vectors can be only in one of two directions, +x or –x, where x represents the coordinate along the track. Since we will be dealing with only two bodies, the Law of Conservation of Momentum can be written as:

( 2 )

Σp_i = Σp_f.

This can be expressed for two bodies as:

( 3 )

m₁v_1ix + m₂v_2ix = m₁v_1fx + m₂v_2fx.

Thus, in order to prove conservation of momentum, we must know the masses of each of the two bodies and their vector velocities (magnitude and direction) before and after the collision. To see if mechanical energy is conserved, we must evaluate the kinetic energy before and after the collision. There is no change in the gravitational potential energy in this case because the motion takes place on a level surface and therefore h — height — does not change. In this case, the conservation of mechanical energy can be expressed with the following equation:

( 4 )

m₁v_1i² +

m₂v_2i² =

m₁v_1f² +

m₂v_2f².

In this lab, you will investigate the type of collisions for which the Law of Conservation of Energy holds true.

Procedure

Please print the worksheet for this lab. You will need this sheet to record your data.

The experimental setup is depicted in fig.1 and 2. The two photogates will record the position of carts as a function of time. This is done by using picket fences of known band spacing.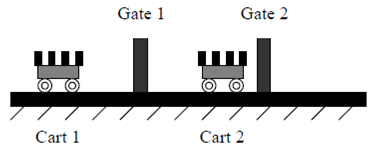

Figure 2

Make sure that the photogate light beam is level with the 1 cm-spaced bands. Open the pre-set experiment file: Labs/PHY 113/PreSetUp Labs/Conservation of Momentum. The recording has to be started and stopped manually.

The carts used in this activity have two kinds of ends. One set of ends allows the carts to stick together (interlocking velcro tape), while the other set of ends, equipped with strong magnets, causes the carts to repel each other.

Before performing the lab, you need to check if the frictionless track is leveled.

The space between the photogates should be wide enough that the first cart collides with the second cart after it completely passes the first photogate. Before each collision, place the second cart right in front of the second photogate. The first cart has to be launched from left to right in the positive x-axis direction.

To achieve the objective of this lab, you have to analyze the following four cases (two inelastic collisions and two elastic collisions).

A
Inelastic collision (carts stick together after the collision), where
- •
  m₁ = m₂
- •
  
  v_2i = 0
B
Inelastic collision (carts stick together after the collision), where
- •
  m₁ ≠ m₂
- •
  
  v_2i = 0
C
Elastic collision (carts stay separated after the collision), where
- •
  m₁ = m₂
- •
  
  v_2i = 0
- •
  The first cart is expected to come to a complete stop after the collision.
D
Elastic collision (carts stay separated after the collision), where
- •
  m₁ < m₂
- •
  
  v_2i = 0
- •
  To significantly change the mass of the cart, add the heavy metal block.
- •
  The first cart is expected to bounce off with a reduced velocity. Be sure to measure and record the velocity of the first cart after the collision, too.

To test if the Law of Conservation of Momentum and the Law of Conservation of Energy hold true in the collisions of the carts, you need to record the mass of each cart, as well as the initial and final velocity of each cart before and after collision. The two graphs provide the data for each cart.

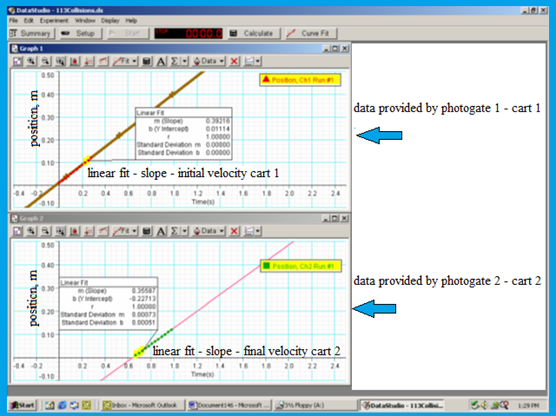
Figure 3: A sample of the experiment file in DataStudio^®

Because the track and the carts are not totally frictionless, it is recommended to fit only a small part of the recorded position closest at the instant of collision. Tabulate the initial velocity of each cart before collisions, and final velocity of each cart after the collision. Note that when the carts stick together and move together after the collision, the velocity of both carts is the same.

Based on recorded data, calculate the momentum of each cart before and after collision. Then, calculate the total momentum of both carts before and after collision. Calculate the percent discrepancy between initial and final momentum of the system. What percent of the momentum has been lost due to friction?

To check if the energy is conserved, calculate the initial and final kinetic energy for each cart. Then, calculate the kinetic energy for both carts before and after the collision. Calculate the percent discrepancy between initial and final energy of the system. In what type of collision is the higher percent of energy lost?

Upload the file with your graphs. Do a print screen and save the graphs as a file with a maximum size of 1 MB. (You will upload this file in the WebAssign question.) Print the graph for your TA to sign, and for your reference.

Discussion

Start your discussion with the statement of the purpose of the lab experiment, then provide a brief theoretical explanation about expected results. Discuss if the graphs generated in class show the expected relationship between the physical quantities. Report your experimental results with their error. Discuss if the theoretical results fit within the error of the experimental results. What is the percent discrepancy (difference) between the theoretical and experimental results? What is the relative error in the experimental results? Are the results accurate and/or precise? In general, the experimental results are considered accurate if the percent discrepancy/difference is within 10-15% depending on the experimental setup used to prove the theoretical concept. The experimental results are considered to be precise if the relative uncertainty is less than 5-10% depending on the experimental setup used to prove the theoretical concept. What are the reasons for random and/or systematic errors? How can they be reduced in the experiment? We are interested primarily in shortcomings in measurement techniques and not in your personal errors (i.e. mistakes). However, if a particular method encourages personal errors, you should describe this as well. The following questions need to be a part of your discussion section. Based on the results you got for the relative percent error, was the momentum conserved in each of the collisions? If not, explain what can be a reason. Based on the results you got for the relative percent error, was the kinetic energy conserved in each of the collisions? If not, explain what can be a reason. In inelastic collisions, there is a more than 60% loss of kinetic energy. What type of energy does the loss of kinetic energy convert into? In general, what collisions are considered to be inelastic and which one is considered to be elastic? Applying these definitions to your data, what type of collisions did the cart experience in the lab? Suggest the way to modify the experiment to reduce the value of the relative change in momentum for inelastic collisions.

Conclusion

Provide a clear statement if the objective of the Lab "Conservation of Momentum and Energy" is met. Do your experimental results confirm the validity of the Law of Conservation of Momentum? Based on your experimental data, did the Law of Conservation of Mechanical Energy hold true in all types of collisions? Support all your answers with evidence.