Angular Momentum

Topics and Files

Mechanics Topics

Rotational motion; position, velocity, acceleration
Rotational motion; velocity versus time

Capstone Files

39 Rotational Motion.cap
40 Rotational Inertia.cap

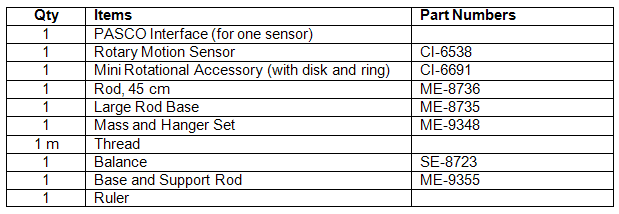
Equipment List

Introduction

This lab has two parts. The purpose of Experiment 1 is to measure the angular position and velocity of a rotating body. Use a rotary motion sensor to measure the rotation of a disk as the disk undergoes a constant angular acceleration. Use Capstone to record and display the data. Plot the angular position and angular velocity and analyze them. Compare the plots of angular position and angular velocity for the accelerating disk to plots of position and velocity for an accelerating fan cart. The purpose of Experiment 2 is to measure the initial and final angular speed of a system consisting of a non-rotating ring that is dropped onto a rotating disk and to verify the conservation of the angular momentum of the system. Use Capstone to record and display the linear speed before and after the torque-free collision.

Background

For each kinematic quantity (i.e. displacement, velocity, etc.) there is an analogous quantity in rotational kinematics. The rotational version of position (x) is the "angular position" that is given by the Greek letter theta. The rotational version of velocity (v) is "angular velocity" that is given by the Greek letter omega. All translational (linear) quantities have rotational counterparts. The equations of kinematics for constant linear acceleration can be used for solving problems involving linear motion in one and two dimensions. For example, the motion of a fan cart accelerating on a flat track can be described by the equations of translational kinematics.

( 1 )

v = v₀ + at

( 2 )

x =

(v₀ + v)t

( 3 )

x = v₀t +

at²

( 4 )

v² = v₀² + 2ax

The ideas of angular displacement, angular velocity, and angular acceleration can be brought together to produce a set of equations called the equations of kinematics for constant angular acceleration.

( 5 )

ω = ω₀ + αt

( 6 )

θ =

(ω₀ + ω)t

( 7 )

θ = ω₀t +

αt²

( 8 )

ω² = ω₀² + 2αθ

The equations of kinematics for constant angular acceleration can be used for solving problems involving rotational motion. For example, the motion of the blades on a fan cart as they start to rotate faster and faster can be described by the equations of rotational kinematics. For rotational motion we defined the torque as analogous quantity to force in translational motion.

( 9 )

τ = Iα

Torque is defined relative to the axis of rotation.

( 10 )

= R

× F

When a net torque τ is applied to an object that is free to rotate, there is a change in the angular momentum (ΔL) of the object.

( 11 )

Δ L

Δt

When a non-rotating disk is dropped on a rotating disk, there is no net torque on the system since the torque on the non-rotating is equal and opposite to the torque on the rotating disk. If there is no change in angular momentum the angular momentum is conserved.

( 12 )

= I_i ω

_i = I_f ω

where

I_i and I_f

are the initial and final rotational inertia of the system, with

_i and ω

being the initial and final rotational speed of the system. The initial rotational inertia is that of a disk and the final inertia is that of the disk and a ring. The ring has a moment of inertia

( 13 )

I =

R₁² + R₂²

where M is the mass of the ring, R₁ is its inner radius, and R₂ the outer radius. The inertia of a solid disk of uniform density is given by

( 14 )

I =

MR²

where M is the mass of the disk and R is its radius.