Lab 4 - Calorimetry
Purpose
To determine if a Styrofoam cup calorimeter provides adequate insulation for heat transfer measurements, to identify an unknown metal by means of its heat capacity and to determine a heat of neutralization and a heat of solution.Goals
-
1To perform simple calorimetry experiments.
-
2To use calorimetry results to calculate the specific heat of an unknown metal.
-
3To determine heat of reaction (ΔH) from calorimetry measurements.
Introduction
Heat and work are the two most common ways for a system to exchange energy with its surroundings. The work term in reactions that do not involve gases is zero, so all of the energy change results in heat. The amount of heat that flows into or out of the surroundings is determined with a technique called calorimetry (heat measurement). A calorimeter is composed of an insulated container, a thermometer, a mass of water, and the system to be studied. The use of an insulated container (Styrofoam cup in this experiment) allows us to assume that there is no heat transferred through the calorimeter walls. In other words, we can assume that the thermodynamic universe is composed of the system and the surrounding water. In the experiment, we will test this assumption. All of the heat absorbed or given off by a system (qsys) is exchanged with its surroundings (qsur), which is expressed mathematically in equation 1.( 1 )
qsys = −qsur
( 2 )
qsur = qwater
( 3 )
qwater = Cwater· ΔTwater
( 4 )
qwater = mwater · swater · ΔTwater
( 5 )
qsys = -qwater = -mwater·swater·ΔTwater = -mwater·(4.18 J/g·°C)·ΔTwater( 6 )
mwarm water swarm water ΔTwarm water = −mcold water scold water ΔTcold water
( 7 )
mmetal smetal ΔTmetal = −mwater swater ΔTwater
( 8 )
NH3(aq) + H3PO4(aq)  NH4+(aq) + H2PO4-(aq)
NH4+(aq) + H2PO4-(aq)( 9 )
NH4H2PO4(s)  NH4+(aq) + H2PO4-(aq)
NH4+(aq) + H2PO4-(aq)( 10 )
ΔH = -mwater swater ΔTwater
( 11 )
| 500 J |
| 0.20 mol |
| J |
| mol |
Equipment
-
1400 mL beaker
-
2Styrofoam cups with lid
-
118 x 150 mm test tube
-
1test tube holder
-
1150 mL beaker
-
1250 mL beaker
-
150 mL beaker
-
2100 mL graduated cylinders
-
1hot plate
-
1MicroLab Interface
-
1MicroLab Thermistor Instruction Sheet
-
1thermistor
-
1deionized water squirt bottle
Reagents
-
~20 gmetal unknowns
-
~50 mL1.5 M NH3
-
~50 mL1.5 M H3PO4
-
~10 gNH4H2PO4
- deionized water
Safety
Phosphoric acid is corrosive. It can attack the skin, cause permanent damage to the eyes, and cause burns. If contact with skin or clothing occurs, immediately rinse the affected area with water for at least 15 minutes and have your lab partner notify the teaching assistant. Do not inhale the fumes. If you inhale enough ammonia vapors to cause discomfort, get to fresh air. Have your lab partner notify the teaching assistant of the problem. Students will have access to gloves owing to the use of concentrated acid and base solutions during the lab period.Waste Disposal
All solutions may be disposed of down the sink drain followed by flushing with plenty of water. Do not put the metal samples in the sink. Return them to the side shelf in the appropriate containers.Prior to Class
Please review the following video: Please complete WebAssign prelab assignment. Check your WebAssign Account for due dates. Students who do not complete the WebAssign prelab are required to bring and hand in the prelab worksheet.Lab Procedure
Please print the worksheet for this lab. You will need this sheet to record your data.Part A: Validating the Assumption about Insulation
1
Start the MicroLab Interface and calibrate the thermistor as described in the MicroLab instructions provided in the lab.
2
Set the MicroLab collection increment to 5 seconds, using the detailed instructions provided in the lab.
3
Measure about 40 mL of deionized water with a graduated cylinder, and add it to a 150 mL beaker. Heat to between 60 and 70°C. Work on steps 4 and 5 while the water is heating.
4
Weigh and record the mass of two nested Styrofoam cups and cover in Data Table A.
Table A: Validating the Assumption about Insulation
5
Measure about 60 mL of deionized water with a graduated cylinder, and add it to the cups. Reweigh the cups, lid, and water, and record this mass in Data Table A. Calculate and record the mass of the "cold water."
6
Set up your calorimeter as follows: place the nested cups in a 400 mL beaker to prevent spilling the contents. Place the lid tightly on the cup.
7
When the warm water is between 60 and 70°C, you are ready to measure temperatures. Insert the thermistor in the warm water; start MicroLab data collection. Measure the temperature of the warm water and record it in Data Table A.
8
Measure the temperature of the water in the calorimeter (cold water) by inserting the thermistor through the hole in the cup lid. When the lid is on the cup, the thermistor should not touch the cup's sides or bottom. Keep the lid tightly on the cup during measurements so all the heat exchange occurs within the cup. Record this temperature in Data Table A.
9
Uncover the calorimeter and quickly pour the warm water into it. Be careful not to let the water spatter from the cup, or you will lose accuracy. Replace the calorimeter cover. Swirl the water carefully so as not to splash or spill the water. Record the temperature of the mixture in Data Table A as soon as it becomes steady, about 30 seconds after mixing.
10
Reweigh the assembly and record the mass in Data Table A. Calculate and record the mass of the warm water you added.
Question 1: When the cold and warm water are mixed, do you expect ΔTcold water and ΔTwarm water to have the same value or different values? What about qcold water and qwarm water?
Question 2: Calculate ΔTcold water, ΔTwarm water, qcold water, and qwarm water. Show your work.


Part B: Identifying an Unknown Metal by Specific Heat
1
Make a boiling water bath with 100 - 150 mL of water in a 250 mL beaker on the hot plate. While it heats, proceed with the following steps.
2
Record the number of the unknown metal you are using in Data Table B.
3
Weigh and record the mass of the unknown metal in Data Table B.
4
Place the metal into a test tube and place the test tube into the 250 mL beaker containing the boiling water.
5
Empty and dry the calorimeter from Part A, then add about 40 mL of water to the calorimeter.
6
Weigh and record the mass of the cups, cover and water in Data Table B. Calculate and record the mass of the water.
7
While the metal is continuing to heat, answer the following questions.
Question 3: Confer with your lab partner and instructor. In this experiment, what is the system and what are the surroundings?
Question 4: When the metal and water are mixed, do you expect ΔTmetal and ΔTwater to be the same or different?
Question 5: When the metal and water are mixed, do you expect qmetal and qwater to be the same or different?
8
Measure the temperature of the water in the calorimeter and record it in Data Table B.
9
After the metal has been in boiling water for 5 - 6 minutes, it is safe to assume the metal is at the temperature of the water. Measure this temperature and record it in Data Table B.
10
Using a test tube holder to avoid being burned, remove the test tube and carefully but quickly pour the metal into the calorimeter. Quickly replace the cover. Be careful not to let water spatter from the cup. Swirl the water gently.
11
Read the temperature of the mixture as soon as it becomes steady and record it in Data Table B.
Table B: Identifying Unknown Metal by Specific Heat
Question 6: Calculate the specific heat of the metal. Show your work.
Question 7: What is the identity of the metal? Refer to the table below.
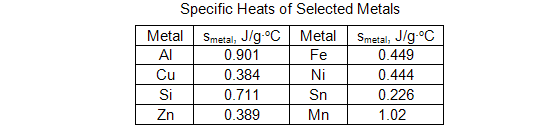

Part C: Heat of Neutralization
1
Empty and dry the calorimeter from Part B.
2
Measure exactly 50.0 mL of 1.50 M NH3 with a graduated cylinder and add the solution to the empty, dry calorimeter.
3
Measure exactly 50.0 mL of 1.50 M H3PO4 into a different graduated cylinder.
4
Cover the calorimeter and insert the thermistor. Measure the initial temperature and record this value in Data Table C. The NH3 solution should be at the same temperature as the H3PO4
solution, thus, one reading will serve as the initial temperature for both.
5
Lift the cover of the calorimeter, add the acid and quickly replace the cover. Swirl gently. When the temperature stops changing (shortly after mixing), record this temperature as the temperature of the mixed solution in Data Table C.
6
Weigh and record the mass of the cups, cover and solution in Data Table C. Calculate the mass of the mixed solution and the phosphoric acid solution.
Table C: Heat of Neutralization
Question 8: What is ΔH for this reaction? Show your work.
Question 9a: Using volume and concentration, how many moles of
NH3
and H3PO4 were mixed together?
Question 9b: What is the molar value for ΔH for this reaction? Show your work.
Question 10 Calculate the amount of NH4H2PO4 that you made in Part C in grams.
Part D: Heat of Solution
1
After thoroughly rinsing and drying your calorimeter, measure 100.0 mL of deionized water in a clean graduated cylinder and add this to the calorimeter.
2
Record the mass of the apparatus plus the water in Data Table D. Calculate the mass of water in the calorimeter.
Table D: Heat of Solution
3
Tare a clean, dry 50 mL beaker. Weigh the amount of NH4H2PO4 you calculated in Question 10 into the 50 mL beaker, and record the exact mass you have obtained in Data Table D.
4
Cover the calorimeter, insert the thermistor and record the initial temperature of the water in Data Table D.
5
Lift the cover, dump the solid into the calorimeter and quickly replace the cover. Swirl gently. It may take a minute or two for the solid to dissolve. Continue swirling until the temperature no longer changes. Record this temperature as the temperature of the solution in Data Table D.
6
After your last measurement, choose stop on the screen and close the MicroLab software. Discard your solution down the sink drain and flush it down with plenty of water. Rinse all of your glassware and calorimeter with water, dry it and return it to the set-up area where you found it.
Question 11: Confer with your lab partner and teaching assistant. In this experiment, what is the system and what are the surroundings?
Question 12: How would you expect the molar change in enthalpy (ΔH) for this reaction to compare with the heat of neutralization in Part C? (If you would like to check, calculate it now. You will be asked for the value in the WebAssign postlab exercise.)
7
Before leaving, enter your results in the InLab assignment. If all results are scored as correct, log out. If not all results are correct, try to find the error or consult with your teaching assistant. When all results are correct, note them and log out of WebAssign. The InLab assignment must be completed by the end of the lab period. If additional time is required, please consult with your teaching assistant.

