4.9�Static and Kinetic Frictional Forces
When an object is in contact with a surface, there is a force acting on the object. The previous section discusses the component of this force that is perpendicular to the surface, which is called the normal force. When the object moves or attempts to move along the surface, there is also a component of the force that is parallel to the surface. This parallel force component is called the frictional force, or simply friction.
In many situations considerable engineering effort is expended trying to reduce friction. For example, oil is used to reduce the friction that causes wear and tear in the pistons and cylinder walls of an automobile engine. Sometimes, however, friction is absolutely necessary. Without friction, car tires could not provide the traction needed to move the car. In fact, the raised tread on a tire is designed to maintain friction. On a wet road, the spaces in the tread pattern (see Figure 4.18) provide channels for the water to collect and be diverted away. Thus, these channels largely prevent the water from coming between the tire surface and the road surface, where it would reduce friction and allow the tire to skid.

Surfaces that appear to be highly polished can actually look quite rough when examined under a microscope. Such an examination reveals that two surfaces in contact touch only at relatively few spots, as Figure 4.19 illustrates. The microscopic area of contact for these spots is substantially less than the apparent macroscopic area of contact between the surfaces—perhaps thousands of times less. At these contact points the molecules of the different bodies are close enough together to exert strong attractive intermolecular forces on one another, leading to what are known as “cold welds.” Frictional forces are associated with these welded spots, but the exact details of how frictional forces arise are not well understood. However, some empirical relations have been developed that make it possible to account for the effects of friction.
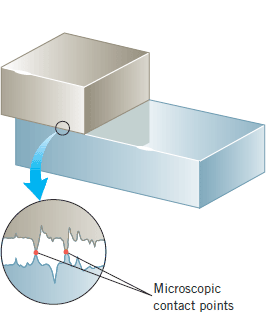
Figure 4.20 helps to explain the main features of the type of friction known as static friction. The block in this drawing is initially at rest on a table, and as long as there is no attempt to move the block, there is no static frictional force. Then, a horizontal force 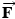 is applied to the block by means of a rope. If
is applied to the block by means of a rope. If 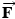 is small, as in part a, experience tells us that the block still does not move. Why? It does not move because the static frictional force
is small, as in part a, experience tells us that the block still does not move. Why? It does not move because the static frictional force  exactly cancels the effect of the applied force. The direction of
exactly cancels the effect of the applied force. The direction of 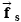 is opposite to that of
is opposite to that of 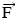 , and the magnitude of
, and the magnitude of 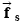 equals the magnitude of the applied force,
equals the magnitude of the applied force,  . Increasing the applied force in Figure 4.20 by a small amount still does not cause the block to move. There is no movement because the static frictional force also increases by an amount that cancels out the increase in the applied force (see part b of the drawing). If the applied force continues to increase, however, there comes a point when the block finally “breaks away” and begins to slide. The force just before breakaway represents the maximum static frictional force
. Increasing the applied force in Figure 4.20 by a small amount still does not cause the block to move. There is no movement because the static frictional force also increases by an amount that cancels out the increase in the applied force (see part b of the drawing). If the applied force continues to increase, however, there comes a point when the block finally “breaks away” and begins to slide. The force just before breakaway represents the maximum static frictional force 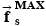 that the table can exert on the block (see part c of the drawing). Any applied force that is greater than
that the table can exert on the block (see part c of the drawing). Any applied force that is greater than 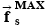 cannot be balanced by static friction, and the resulting net force accelerates the block to the right.
cannot be balanced by static friction, and the resulting net force accelerates the block to the right.

 to the block produces no movement, because the static frictional force
to the block produces no movement, because the static frictional force  exactly balances the applied force. (c) The block just begins to move when the applied force is slightly greater than the maximum static frictional force
exactly balances the applied force. (c) The block just begins to move when the applied force is slightly greater than the maximum static frictional force  .
.Experimental evidence shows that, to a good degree of approximation, the maximum static frictional force between a pair of dry, unlubricated surfaces has two main characteristics. It is independent of the apparent macroscopic area of contact between the objects, provided that the surfaces are hard or nondeformable. For instance, in Figure 4.21 the maximum static frictional force that the surface of the table can exert on a block is the same, whether the block is resting on its largest or its smallest side. The other main characteristic of  is that its magnitude is proportional to the magnitude of the normal force
is that its magnitude is proportional to the magnitude of the normal force 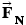 . As Section 4.8 points out, the magnitude of the normal force indicates how hard two surfaces are being pressed together. The harder they are pressed, the larger is
. As Section 4.8 points out, the magnitude of the normal force indicates how hard two surfaces are being pressed together. The harder they are pressed, the larger is  , presumably because the number of “cold-welded,” microscopic contact points is increased. Equation 4.7 expresses the proportionality between
, presumably because the number of “cold-welded,” microscopic contact points is increased. Equation 4.7 expresses the proportionality between 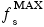 and
and  with the aid of a proportionality constant
with the aid of a proportionality constant 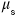 , which is called the coefficient of static friction.
, which is called the coefficient of static friction.

 would be the same, no matter which side of the block is in contact with the table.
would be the same, no matter which side of the block is in contact with the table. | Concept Simulation 4.2: Static Friction |
Static Frictional Force
The magnitude 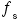 of the static frictional force can have any value from zero up to a maximum value of
of the static frictional force can have any value from zero up to a maximum value of 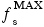 , depending on the applied force. In other words,
, depending on the applied force. In other words, 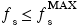 , where the symbol
, where the symbol 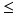 is read as “less than or equal to.” The equality holds only when
is read as “less than or equal to.” The equality holds only when 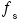 attains its maximum value, which is
attains its maximum value, which is

In Equation 4.7,  is the coefficient of static friction, and
is the coefficient of static friction, and 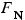 is the magnitude of the normal force.
is the magnitude of the normal force.
It should be emphasized that Equation 4.7 relates only the magnitudes of  and
and 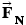 , not the vectors themselves. This equation does not imply that the directions of the vectors are the same. In fact,
, not the vectors themselves. This equation does not imply that the directions of the vectors are the same. In fact, 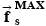 is parallel to the surface, while
is parallel to the surface, while  is perpendicular to it.
is perpendicular to it.
The coefficient of static friction, being the ratio of the magnitudes of two forces 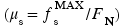 , has no units. Also, it depends on the type of material from which each surface is made (steel on wood, rubber on concrete, etc.), the condition of the surfaces (polished, rough, etc.), and other variables such as temperature. Table 4.2 gives some typical values of
, has no units. Also, it depends on the type of material from which each surface is made (steel on wood, rubber on concrete, etc.), the condition of the surfaces (polished, rough, etc.), and other variables such as temperature. Table 4.2 gives some typical values of 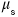 for various surfaces. Example 9 illustrates the use of Equation 4.7 for determining the maximum static frictional force.
for various surfaces. Example 9 illustrates the use of Equation 4.7 for determining the maximum static frictional force.
| Materials | Coefficient of Static Friction, μs | Coefficient of Kinetic Friction, μk |
|---|---|---|
| Glass on glass (dry) | 0.94 | 0.4 |
| Ice on ice (clean, 0�C) | 0.1 | 0.02 |
| Rubber on dry concrete | 1.0 | 0.8 |
| Rubber on wet concrete | 0.7 | 0.5 |
| Steel on ice | 0.1 | 0.05 |
| Steel on steel (dry hard steel) | 0.78 | 0.42 |
| Teflon on Teflon | 0.04 | 0.04 |
| Wood on wood | 0.35 | 0.3 |
Analyzing Multiple-Concept Problems�
EXAMPLE�9The Force Needed to Start a Skier Moving
A skier is standing motionless on a horizontal patch of snow. She is holding onto a horizontal tow rope, which is about to pull her forward (see Figure 4.22a). The skier's mass is 59 kg, and the coefficient of static friction between the skis and snow is 0.14. What is the magnitude of the maximum force that the tow rope can apply to the skier without causing her to move?

When the rope applies a relatively small force, the skier does not accelerate. The reason is that the static frictional force opposes the applied force and the two forces have the same magnitude. We can apply Newton's second law in the horizontal direction to this situation. In order for the rope to pull the skier forward, it must exert a force large enough to overcome the maximum static frictional force acting on the skis. The magnitude of the maximum static frictional force depends on the coefficient of static friction (which is known) and on the magnitude of the normal force. We can determine the magnitude of the normal force by using Newton's second law, along with the fact that the skier does not accelerate in the vertical direction.
Knowns and Unknowns The data for this problem are as follows:
| Description | Symbol | Value |
|---|---|---|
| Mass of skier | m | 59 kg |
| Coefficient of static friction | μs | 0.14 |
| Unknown Variable | � | � |
| Magnitude of maximum horizontal force that tow rope can apply | F | ? |
 Newton's Second Law (Horizontal Direction) Figure 4.22a shows the two horizontal forces that act on the skier just before she begins to move: the force Newton's Second Law (Horizontal Direction) Figure 4.22a shows the two horizontal forces that act on the skier just before she begins to move: the force 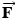 applied by the tow rope and the maximum static frictional force applied by the tow rope and the maximum static frictional force  . Since the skier is standing motionless, she is not accelerating in the horizontal or . Since the skier is standing motionless, she is not accelerating in the horizontal or  direction, so direction, so 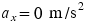 . Applying Newton's second law (Equation 4.2a) to this situation, we have . Applying Newton's second law (Equation 4.2a) to this situation, we have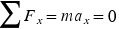  in the in the 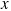 direction is direction is 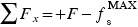 , Newton's second law can be written as , Newton's second law can be written as  . Thus, . Thus,
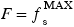 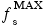 , but its value will be determined in Steps 2 and 3. , but its value will be determined in Steps 2 and 3. |  |
 The Maximum Static Frictional Force The magnitude The Maximum Static Frictional Force The magnitude 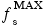 of the maximum static frictional force is related to the coefficient of static friction of the maximum static frictional force is related to the coefficient of static friction 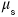 and the magnitude and the magnitude 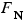 of the normal force by Equation 4.7: of the normal force by Equation 4.7: 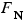 is not. An expression for is not. An expression for  will be obtained in the next step. will be obtained in the next step. | 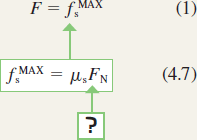 |
 Newton's Second Law (Vertical Direction) We can find the magnitude Newton's Second Law (Vertical Direction) We can find the magnitude  of the normal force by noting that the skier does not accelerate in the vertical or of the normal force by noting that the skier does not accelerate in the vertical or  direction, so direction, so 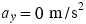 . Figure 4.22b shows the two vertical forces that act on the skier: the normal force . Figure 4.22b shows the two vertical forces that act on the skier: the normal force  and her weight and her weight 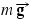 . Applying Newton's second law (Equation 4.2b) in the vertical direction gives . Applying Newton's second law (Equation 4.2b) in the vertical direction gives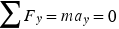  direction is direction is 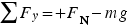 , so Newton's second law becomes , so Newton's second law becomes 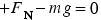 . Thus, . Thus,
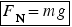 |  |
Algebraically combining the results of the three steps, we have

The magnitude  of the maximum force is
of the maximum force is

If the force exerted by the tow rope exceeds this value, the skier will begin to accelerate forward.
 The physics of rock climbing. Static friction is often essential, as it is to the rock climber in Figure 4.23, for instance. She presses outward against the walls of the rock formation with her hands and feet to create sufficiently large normal forces, so that the static frictional forces help support her weight.
The physics of rock climbing. Static friction is often essential, as it is to the rock climber in Figure 4.23, for instance. She presses outward against the walls of the rock formation with her hands and feet to create sufficiently large normal forces, so that the static frictional forces help support her weight.

Once two surfaces begin sliding over one another, the static frictional force is no longer of any concern. Instead, a type of friction known as kinetic3 friction comes into play. The kinetic frictional force opposes the relative sliding motion. If you have ever pushed an object across a floor, you may have noticed that it takes less force to keep the object sliding than it takes to get it going in the first place. In other words, the kinetic frictional force is usually less than the static frictional force.
Experimental evidence indicates that the kinetic frictional force 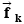 has three main characteristics, to a good degree of approximation. It is independent of the apparent area of contact between the surfaces (see Figure 4.21). It is independent of the speed of the sliding motion, if the speed is small. And last, the magnitude of the kinetic frictional force is proportional to the magnitude of the normal force. Equation 4.8 expresses this proportionality with the aid of a proportionality constant
has three main characteristics, to a good degree of approximation. It is independent of the apparent area of contact between the surfaces (see Figure 4.21). It is independent of the speed of the sliding motion, if the speed is small. And last, the magnitude of the kinetic frictional force is proportional to the magnitude of the normal force. Equation 4.8 expresses this proportionality with the aid of a proportionality constant 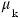 , which is called the coefficient of kinetic friction.
, which is called the coefficient of kinetic friction.
Kinetic Frictional Force
The magnitude 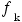 of the kinetic frictional force is given by
of the kinetic frictional force is given by

In Equation 4.8, 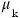 is the coefficient of kinetic friction, and
is the coefficient of kinetic friction, and  is the magnitude of the normal force.
is the magnitude of the normal force.
Equation 4.8, like Equation 4.7, is a relationship between only the magnitudes of the frictional and normal forces. The directions of these forces are perpendicular to each other. Moreover, like the coefficient of static friction, the coefficient of kinetic friction is a number without units and depends on the type and condition of the two surfaces that are in contact. As indicated in Table 4.2, values for  are typically less than those for
are typically less than those for  , reflecting the fact that kinetic friction is generally less than static friction. The next example illustrates the effect of kinetic friction.
, reflecting the fact that kinetic friction is generally less than static friction. The next example illustrates the effect of kinetic friction.
Analyzing Multiple-Concept Problems�
EXAMPLE�10Sled Riding
A sled and its rider are moving at a speed of  along a horizontal stretch of snow, as Figure 4.24a illustrates. The snow exerts a kinetic frictional force on the runners of the sled, so the sled slows down and eventually comes to a stop. The coefficient of kinetic friction is 0.050. What is the displacement
along a horizontal stretch of snow, as Figure 4.24a illustrates. The snow exerts a kinetic frictional force on the runners of the sled, so the sled slows down and eventually comes to a stop. The coefficient of kinetic friction is 0.050. What is the displacement  of the sled?
of the sled?
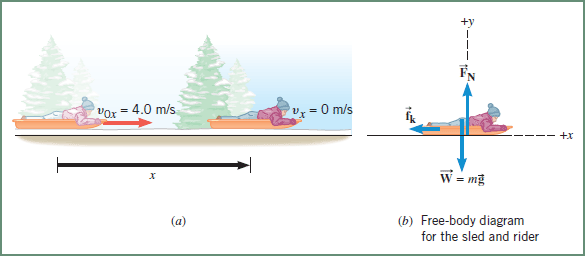
 of the sled and its rider, the normal force
of the sled and its rider, the normal force  , and the kinetic frictional force
, and the kinetic frictional force  . The free-body diagram for the sled and rider shows these forces.
. The free-body diagram for the sled and rider shows these forces.As the sled slows down, its velocity is decreasing. As our discussions in Chapters 2 and 3 indicate, the changing velocity is described by an acceleration (which in this case is a deceleration since the sled is slowing down). Assuming that the acceleration is constant, we can use one of the equations of kinematics from Chapter 3 to relate the displacement to the initial and final velocities and to the acceleration. The acceleration of the sled is not given directly. However, we can determine it by using Newton's second law of motion, which relates the acceleration to the net force (which is the kinetic frictional force in this case) acting on the sled and to the mass of the sled (plus rider).
Knowns and Unknowns The data for this problem are listed in the table:
| Description | Symbol | Value | Comment |
|---|---|---|---|
| Explicit Data | � | � | � |
| Initial velocity | v0x | +4.0 m/s | Positive, because the velocity points in the +x direction. |
| � | � | � | See the drawing. |
| Coefficient of kinetic friction | μk | 0.050 | � |
| Implicit Data | � | � | � |
| Final velocity | vx | 0 m/s | The sled comes to a stop. |
| Unknown Variable | � | � | � |
| Displacement | x | ? | � |
 Displacement To obtain the displacement Displacement To obtain the displacement  of the sled we will use Equation 3.6a from the equations of kinematics: of the sled we will use Equation 3.6a from the equations of kinematics: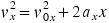  gives the result shown at the right. This equation is useful because two of the variables, gives the result shown at the right. This equation is useful because two of the variables,  and and  , are known and the acceleration , are known and the acceleration 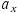 can be found by applying Newton's second law to the accelerating sled (see Step 2). can be found by applying Newton's second law to the accelerating sled (see Step 2). | 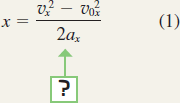 |
 Newton's Second Law Newton's second law, as given in Equation 4.2a, states that the acceleration Newton's Second Law Newton's second law, as given in Equation 4.2a, states that the acceleration  is equal to the net force is equal to the net force  divided by the mass divided by the mass 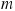 : :  direction is the kinetic frictional force direction is the kinetic frictional force  . We can write this force as . We can write this force as 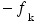 , where , where 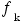 is the magnitude of the force and the minus sign indicates that it points in the is the magnitude of the force and the minus sign indicates that it points in the 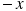 direction. Since the net force is direction. Since the net force is  , Equation 4.2a becomes , Equation 4.2a becomes
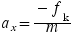 |  |
 Kinetic Frictional Force We do not know the magnitude Kinetic Frictional Force We do not know the magnitude 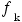 of the kinetic frictional force, but we do know the coefficient of kinetic friction of the kinetic frictional force, but we do know the coefficient of kinetic friction 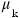 . According to Equation 4.8, the two are related by . According to Equation 4.8, the two are related by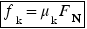 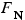 is the magnitude of the normal force. This relation can be substituted into Equation 2, as shown at the right. An expression for is the magnitude of the normal force. This relation can be substituted into Equation 2, as shown at the right. An expression for 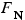 will be obtained in the next step. will be obtained in the next step. |  |
 Normal Force The magnitude Normal Force The magnitude  of the normal force can be found by noting that the sled does not accelerate in the vertical or of the normal force can be found by noting that the sled does not accelerate in the vertical or 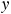 direction direction 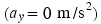 . Thus, Newton's second law, as given in Equation 4.2b, becomes . Thus, Newton's second law, as given in Equation 4.2b, becomes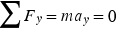 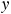 direction: the normal force direction: the normal force 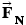 and its weight and its weight 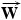 (see Figure 4.24b). Therefore, the net force in the (see Figure 4.24b). Therefore, the net force in the 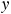 direction is direction is
 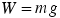 (Equation 4.5). Thus, Newton's second law becomes (Equation 4.5). Thus, Newton's second law becomes
 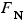 can be substituted into Equation 4.8, as shown at the right. can be substituted into Equation 4.8, as shown at the right. |  |
Algebraically combining the results of each step, we find that

Note that the mass 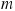 of the sled and rider is algebraically eliminated from the final result. Thus, the displacement of the sled is
of the sled and rider is algebraically eliminated from the final result. Thus, the displacement of the sled is

Static friction opposes the impending relative motion between two objects, while kinetic friction opposes the relative sliding motion that actually does occur. In either case, relative motion is opposed. However, this opposition to relative motion does not mean that friction prevents or works against the motion of all objects. For instance, consider what happens when you walk.
 The physics of walking. Your foot exerts a force on the earth, and the earth exerts a reaction force on your foot. This reaction force is a static frictional force, and it opposes the impending backward motion of your foot, propelling you forward in the process. Kinetic friction can also cause an object to move, all the while opposing relative motion, as it does in Example 10. In this example the kinetic frictional force acts on the sled and opposes the relative motion of the sled and the earth. Newton's third law indicates, however, that since the earth exerts the kinetic frictional force on the sled, the sled must exert a reaction force on the earth. In response, the earth accelerates, but because of the earth's huge mass, the motion is too slight to be noticed.
The physics of walking. Your foot exerts a force on the earth, and the earth exerts a reaction force on your foot. This reaction force is a static frictional force, and it opposes the impending backward motion of your foot, propelling you forward in the process. Kinetic friction can also cause an object to move, all the while opposing relative motion, as it does in Example 10. In this example the kinetic frictional force acts on the sled and opposes the relative motion of the sled and the earth. Newton's third law indicates, however, that since the earth exerts the kinetic frictional force on the sled, the sled must exert a reaction force on the earth. In response, the earth accelerates, but because of the earth's huge mass, the motion is too slight to be noticed.
 |
Concept Simulation 4.3: Stopping Distance of a Car Concept Simulation 4.4: The Normal Force and the Static Frictional Force |
Check Your Understanding�
Suppose that the coefficients of static and kinetic friction have values such that 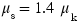 for a crate in contact with a cement floor. Which one of the following statements is true?
for a crate in contact with a cement floor. Which one of the following statements is true?
- (a)The magnitude of the static frictional force is always 1.4 times the magnitude of the kinetic frictional force.
- (b)The magnitude of the kinetic frictional force is always 1.4 times the magnitude of the static frictional force.
- (c)The magnitude of the maximum static frictional force is 1.4 times the magnitude of the kinetic frictional force.
A person has a choice of either pushing or pulling a sled at a constant velocity, as the drawing illustrates. Friction is present. If the angle 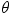 is the same in both cases, does it require less force to push or to pull the sled?
is the same in both cases, does it require less force to push or to pull the sled?

A box has a weight of 150 N and is being pulled across a horizontal floor by a force that has a magnitude of 110 N. The pulling force can point horizontally, or it can point above the horizontal at an angle 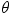 . When the pulling force points horizontally, the kinetic frictional force acting on the box is twice as large as when the pulling force points at the angle
. When the pulling force points horizontally, the kinetic frictional force acting on the box is twice as large as when the pulling force points at the angle 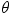 . Find
. Find 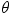 .
.
- (a)stationary,
- (b)accelerating upward, and
- (c)accelerating downward. Rank the forces required in these three situations in ascending order—that is, smallest first.

