Speed of Sound - Resonance Tube

Objectives

•
to determine the speed of sound waves in air

Equipment

resonance tube, tuning forks, rubber mallet, measuring tape, thermometer

Figure 1

Figure 2

Introduction and Theory

A sound wave is a longitudinal wave in which the wave oscillates along the direction of propagation. For a traveling wave of speed v, frequency f, and wavelength λ, the following relationship holds.

( 1 )

v = fλ

In this lab, we are going to use a simple characteristic of the traveling wave—the resonance—to determine the wavelength (and therefore the speed) of a sound wave. Consider a sound wave traveling through a resonance tube as illustrated in Fig. 3.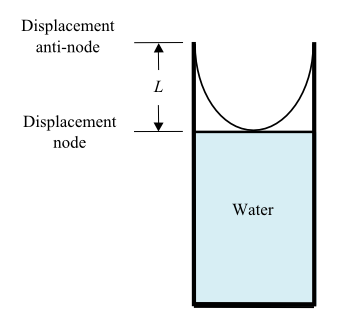

Figure 3: Resonance tube

A tuning fork is held by hand just above the open end of the tube. When the tuning fork is struck by a rubber hammer, it vibrates, and sound waves are generated. These sound waves travel down the tube and are reflected upon reaching the surface of the water. The incoming and reflected waves interfere and form standing waves. The sound waves reflected from the water surface change their phase by 180° and therefore are completely out of phase with the incident sound waves. In other words, the amplitude of the standing waves must be zero at the water's surface. This point in space is usually referred to as a node. If a resonance condition is met, the open end of the tube has maximum amplitude of standing sound waves and is called an antinode. A standing wave called a vibrational pattern is created within a medium when the vibrational frequency of the source causes reflected waves from one end of the medium to interfere with incident waves from the source. Incident and reflected waves are waves of equal amplitude, frequency, and wavelength. The wave pattern does not appear to be moving in either direction and is confined within the boundaries. At constant temperature, the speed of sound is fixed; in addition, for a given tuning fork the frequency is also fixed, so according to equation 1

v = fλ

, the wavelength of the sound wave should also be fixed. As a result, the resonance conditions can only be satisfied when the length of the tube L is such that

( 2 )

L_n =

(2n + 1)λ,

where n = 0, 1, 2, 3, 4,..., and the length L_n is defined to be the distance measured from the open end of the tube to the water surface. For the specific example given in Fig. 3, the first resonance is shown, when n = 0, and from equation 2, the length of the tube is

L =

λ.

Fig. 4 shows resonance conditions in which n = 1, 2, and 3 (resonance 2, 3, and 4). It is easy to notice that

L₂ − L₁ =

and L₃ − L₂ =

λ.

It can be predicted that the next resonance will occur at

L₄ =

λ +

λ =

λ.

In other words, we have the following equations.

( 3 )

L_{n + 1} − L_n =

( 4 )

ΔL =

This relationship between the two consecutive resonances will be used to find the wavelength of the standing sound wave. The objective of this lab is to measure the speed of a sound wave in the air and compare it to its theoretical value.

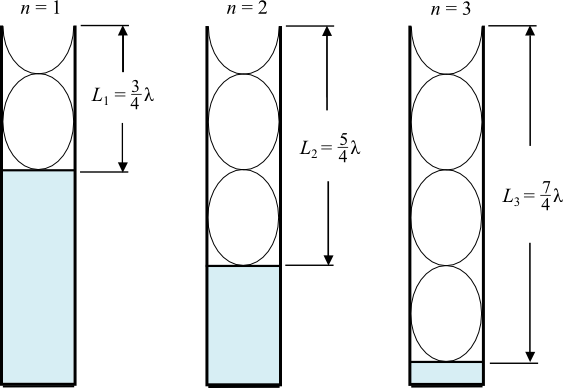
Figure 4: Examples of resonance for n = 1, 2, and 3

According to the theory, the speed of sound in air depends upon the temperature of the air through the following relationship.

( 5 )

v_T = (331.5 + 0.606T) m/s

Here, T is the temperature in centigrade (degrees Celsius). To achieve the objective, your TA will provide you with two tuning forks of known frequencies f. Remember to return both tuning forks to your TA by the end of the session. Be sure you clean your working space after you complete the experiment.

Video

View the video below prior to beginning your lab.

Procedure

Please print the worksheet for this lab. Answer all the questions on the Lab Worksheet prior to submitting the Inlab in WebAssign.

Checkpoint:
Be sure to have your TA sign your lab worksheet, printed Inlab, and all printed graphs after each part is completed. Be sure the data can be seen on the graphs.

Watch the level of water in the side container to avoid overflowing. Be sure to clean up any spills.

You will be provided with two tuning forks of known frequencies f. Note that the frequencies of the tuning forks are marked on them. Record them in the Inlab in WebAssign.

Fill the tube with water to about 10 cm from the open end of the tube. The level of the water in the tube (= the length of the tube L) can be adjusted by moving the side bucket up and down in the vertical direction.

Strike the tuning fork with the rubber head of the mallet for forks with frequencies below

1000 Hz

or with the wooden head for forks with frequencies above 1000 Hz, and place it just above the open end of the tube. Neither the hammer nor the vibrating fork should touch the tube.

Find as many resonances as you can for the first tuning fork. Repeat your measurements by increasing the water level in the tube. Measure the length of the air column for each resonance from the top edge of the tube. Find the difference in length (ΔL) between the two consecutive resonances to calculate the wavelength of the sound wave. Once the wavelength is determined, the speed of sound follows from equation 1

v = fλ

. (Note that the error in the measurement of the length is 1 mm.)

Repeat steps 3–4 for the other tuning fork with a different frequency. In the Inlab, you will need to calculate the average of the two measured speeds of sound and compare them to the theoretical value.

Adapted from PASCO scientific at pasco.com.